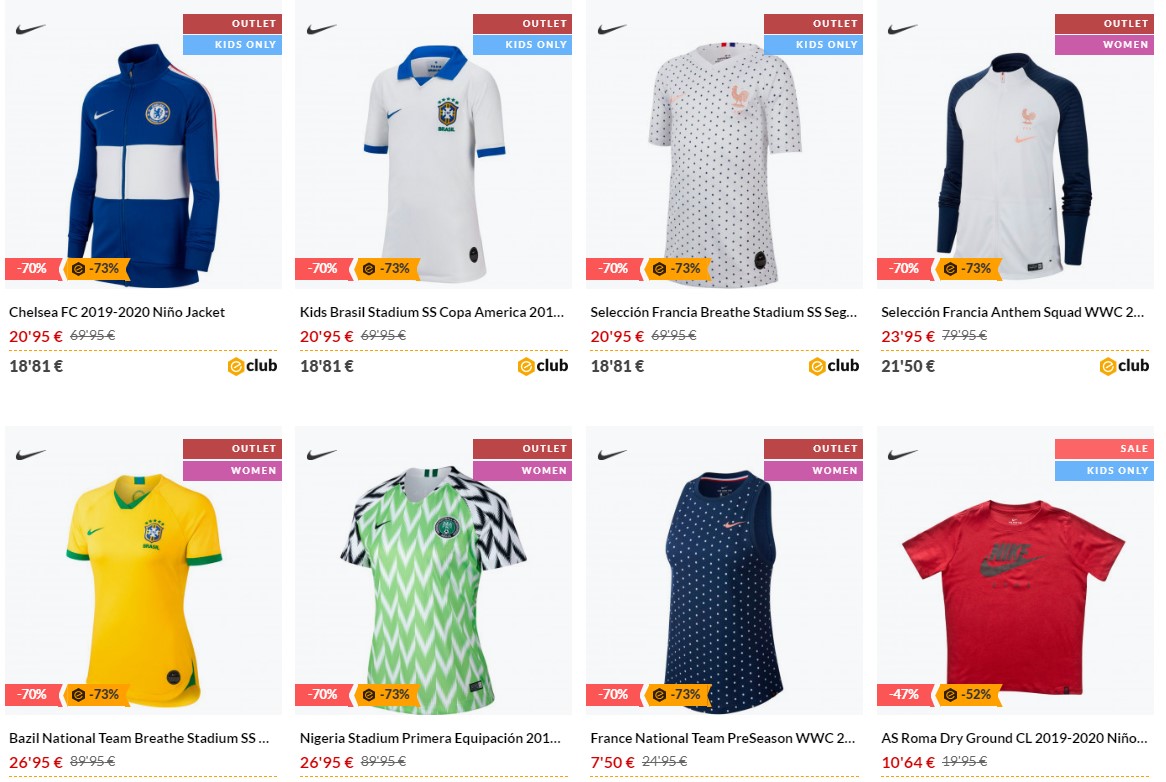
24-25 paginas camisetas futbol baratas
Te presentamos las mejores casas de apuestas para que veas partidos de fútbol en streaming. Barcelona y Alavés se enfrentan hoy domingo 12 de noviembre a las 16:15 horas en el Estadio Olímpico de Montjuic en el partido correspondiente a la jornada 13 de LaLiga EA Sports. FC Barcelona y Deportivo Alavés se enfrentan en un duelo aparentemente desigual en la 13º jornada de LaLiga EA Sports. Ahora mismo el FC Barcelona se encuentra 3º con 27 puntos. Free Hit: Permite cambiar el equipo por completo sin coste en puntos. La selección de fútbol de Australia (en inglés: Australian national soccer team) es el equipo masculino representativo de ese país para la práctica de este deporte. La Selección española encara su segundo y último partido de esta fecha FIFA. Copa del Mundo de la FIFA. En las eliminatorias para la Copa Mundial de Fútbol de 2018, la selección de Polonia fue encuadrada en el grupo E, junto con Dinamarca, Armenia, Kazajistán, Montenegro y Rumania. El partido de Liga entre Estadio Olímpico de Montjuic de la jornada 13 de LaLiga EA Sports se disputa hoy en el Estadio Olímpico de Montjuic a las 16:15 horas y se podrá ver por televisión en directo a través del canal Movistar LaLiga TV.
Vive la pasión del fútbol y disfruta de LALIGA EA SPORTS y LALIGA HYPERMOTION, así como la Champions League, UEFA Europa League. Recibe en su estadio a un SK Brann que terminó como segundo clasificado del Grupo B con 13 puntos, camiseta celta 2024 por detrás del Olympique de Lyon femenino. ¿Dónde ver el el Barça femenino – SK Brann? ¿Dónde ver en TV el España – Brasil? El amistoso entre España y Brasil se disputa el martes 26 de marzo a las 21:30 hora española. ¿A qué hora es el España – Brasil? Posteriormente, Costa Rica ganó en Puerto España a Trinidad y Tobago por 2 a 0 con dos goles de Rónald Gómez y a Estados Unidos por 2 a 0 en el Ricardo Saprissa con dos goles de Rolando Fonseca y se empató ante México a cero goles en el Saprissa. España ha ganado con solvencia los cinco encuentros anteriores: 3-1 contra Georgia, 1-3 frente a Chipre, 0-1 ante la Noruega de Haaland, 2-0 contra Escocia y un contundente 6-0 a Chipre de nuevo. Los últimos seis enfrentamientos entre FC Barcelona y Deportivo Alavés se han saldado con 4 victorias culés (4-1, 0-5, 5-1 y 0-1) y dos empates (ambos por 1-1). Para encontrar la última victoria del Alavés ante el FC Barcelona nos tenemos que remontar a la tercera jornada de LaLiga 2016/2017, cuando los vascos vencieron 1-2 a los catalanes.
Los suizos fueron eliminados de los Juegos Olímpicos de Ámsterdam 1928 después de derrotar a Alemania por 4-0. Los resultados no fueron mejores en la Copa Internacional, el predecesor del Campeonato Europeo, donde Suiza ocupó el último lugar en las seis ediciones. Así pues, y reforzado el conjunto blaugrana tras golear al Real Madrid femenino el pasado domingo en la Liga F (0-3), las de Jonathan Giráldez esperan dar su mejor versión en el estadio Johan Cruyff. ¿Cuándo se juega el Barça femenino – SK Brann? El Barça quiere seguir con su claro dominio del fútbol femenino europeo. Alineación probable del FC Barcelona: Ter Stegen; Araújo, Koundé, Iñigo, Balde; Gündogan, Pedri, Fermín López; Raphinha, Lewandowski, Joao Félix. Alineación probable del Alavés: Sivera; Gorosabel, Abqar, Rafa Marín, Javi López; Antonio Blanco, Guridi, Guevara; Hagi, Kike García, Luis Rioja. La convocatoria del chico de 17 años del Fútbol Club Barcelona ha levantado mucha expectativa, y ya debutó ante Colombia jugando los últimos minutos del partido. Al final de los años Noventa, debido a graves problemas de salud del presidente Caminiti, la Federación dejó de hacer actividad agonista y se mantuvo latente hasta el verano de 2009 cuando, con la llegada de Axel Paderni, retomó la actividad deportiva.
Bienvenido a La Casa del Fútbol. En el partido de ida ante el SK Brann Kvinner, los goles de Caroline Graham Hansen y de Salma Paralluelo dieron la ventaja el Barça, que confía en pasar por encima de su rival al contar con el calor de su afición en el estadio de fútbol Johan Cruff. Himno nacional de Grecia que se escucha en el estadio antes de los partidos de la selección. Por lo que respecta a las competiciones de los clubes, la FIFS inscribió a la Copa de Campeones para Clubes a los equipos que ganaban la liga nacional del 1988 al 1998: en la edición del 1991 la A.S. Su primera participación efectiva fue en el Campeonato Nacional 1973, como club indirectamente afiliado a la AFA, a través de la plaza fija de la Liga Tucumana. También se volvió usual la impresión del logotipo de patrocinadores tanto en camisetas como pantalones. Era meticuloso y le gustaba estudiar al contrario tanto que un día que el Atlético de Madrid jugaba en Oviedo Falín acudió a la estación a esperar a los jugadores. El FC Barcelona buscará seguir manteniéndose en lo alto de la clasificación, pero ojo que el Real Madrid no dará su brazo a torcer.




 Los datos apuntan a que cada vez más jugadores prefieren demorar su reincorporación a cambio de hacerlo con mayores garantías de evitar recaídas. Para ello obtiene datos que los clubes ofrecen directamente a la UEFA y que Ekstrand debe tratar con la más absoluta discreción. El Confidencial, «y eso es porque a veces lo que los clubes cuentan no refleja la verdadera situación». El Confidencial. «A veces, lo que los clubes reportan a los medios de comunicación no refleja la situación real». No hay ningún partido final, que es un tema muy controvertido. Sin embargo, siempre hay un factor de azar, y por eso los investigadores suelen medir esto en minutos de exposición: a más tiempo sobre el césped (entrenando o jugando, aunque las posibilidades de lesionarse en un partido son mucho mayores) más posibilidad de lesionarse. Esto lleva generando debates en la prensa deportiva desde hace más de medio siglo. De hecho, hay jugadores contemporáneos que han tenido tiempos de recuperación similares a los que tuvo Camacho: Jermaine Jenas, antiguo internacional inglés, pasó 619 días convaleciente entre 2014 y 2016 hasta que, dada su edad, decidió no seguir intentándolo y se retiró. Ádám Bogdán, eterno suplente del Liverpool, estuvo 587 días en el dique seco entre 2016 y 2017. Giuseppe Rossi, ex del Manchester United o el Villarreal, pasó 557 días recuperándose del cruzado roto y logró volver por sus fueros, de hecho, se retiró el pasado verano a los 36 años.
Los datos apuntan a que cada vez más jugadores prefieren demorar su reincorporación a cambio de hacerlo con mayores garantías de evitar recaídas. Para ello obtiene datos que los clubes ofrecen directamente a la UEFA y que Ekstrand debe tratar con la más absoluta discreción. El Confidencial, «y eso es porque a veces lo que los clubes cuentan no refleja la verdadera situación». El Confidencial. «A veces, lo que los clubes reportan a los medios de comunicación no refleja la situación real». No hay ningún partido final, que es un tema muy controvertido. Sin embargo, siempre hay un factor de azar, y por eso los investigadores suelen medir esto en minutos de exposición: a más tiempo sobre el césped (entrenando o jugando, aunque las posibilidades de lesionarse en un partido son mucho mayores) más posibilidad de lesionarse. Esto lleva generando debates en la prensa deportiva desde hace más de medio siglo. De hecho, hay jugadores contemporáneos que han tenido tiempos de recuperación similares a los que tuvo Camacho: Jermaine Jenas, antiguo internacional inglés, pasó 619 días convaleciente entre 2014 y 2016 hasta que, dada su edad, decidió no seguir intentándolo y se retiró. Ádám Bogdán, eterno suplente del Liverpool, estuvo 587 días en el dique seco entre 2016 y 2017. Giuseppe Rossi, ex del Manchester United o el Villarreal, pasó 557 días recuperándose del cruzado roto y logró volver por sus fueros, de hecho, se retiró el pasado verano a los 36 años. 45 años antes que Gavi, una mañana de enero de 1978, un fulgurante lateral zurdo del Real Madrid llamado José Antonio Camacho se rompía el mismo ligamento cruzado. Ahí están ejemplos como Xavi, que se sobrepuso a una justo antes de convertirse en el diapasón de la España del tikitaka, Sergio Canales que pasó hasta tres veces por quirófano y acabó siendo una leyenda en el Betis o Zlatan Ibrahimović, que se acabó retirando el verano pasado a los 41 años. Sin embargo, no cabe duda de que hoy en día una lesión de cruzado no supone el final de un jugador. En la última década y media, se han registrado más de 2.000 lesiones relacionadas con el ligamento cruzado entre los futbolistas que han participado en alguna de las cinco grandes ligas. La plétora de lesiones graves en futbolistas mediáticos ha llevado a entrenadores como Guardiola a denunciar lo sobrecargado del calendario, ¿pero es cierto que esté habiendo cada vez más lesiones? En general las lesiones se han reducido, debido sobre todo a los progresos médicos y técnicos, que permiten medir mejor el esfuerzo de los futbolistas o personalizar sus entrenamientos al milímetro. También ha sido galardonado por partida doble su compañero de equipo, el portero Marc André ter Stegen con el Premio MARCA Zamora al guardameta menos goleado y el Trofeo Alfredo di Stéfano, que reconoce al mejor jugador del campeonato.
45 años antes que Gavi, una mañana de enero de 1978, un fulgurante lateral zurdo del Real Madrid llamado José Antonio Camacho se rompía el mismo ligamento cruzado. Ahí están ejemplos como Xavi, que se sobrepuso a una justo antes de convertirse en el diapasón de la España del tikitaka, Sergio Canales que pasó hasta tres veces por quirófano y acabó siendo una leyenda en el Betis o Zlatan Ibrahimović, que se acabó retirando el verano pasado a los 41 años. Sin embargo, no cabe duda de que hoy en día una lesión de cruzado no supone el final de un jugador. En la última década y media, se han registrado más de 2.000 lesiones relacionadas con el ligamento cruzado entre los futbolistas que han participado en alguna de las cinco grandes ligas. La plétora de lesiones graves en futbolistas mediáticos ha llevado a entrenadores como Guardiola a denunciar lo sobrecargado del calendario, ¿pero es cierto que esté habiendo cada vez más lesiones? En general las lesiones se han reducido, debido sobre todo a los progresos médicos y técnicos, que permiten medir mejor el esfuerzo de los futbolistas o personalizar sus entrenamientos al milímetro. También ha sido galardonado por partida doble su compañero de equipo, el portero Marc André ter Stegen con el Premio MARCA Zamora al guardameta menos goleado y el Trofeo Alfredo di Stéfano, que reconoce al mejor jugador del campeonato. Pero el fútbol moderno , el que nos entretiene todos los fines de semana (y entre semana), se constituyó a mediados del siglo XIX, cuando se fue reglamentando un juego que practicaban estudiantes en los pueblos y los colegios británicos según sus propias reglas, y del que, con otras reglas, también nació el Rugby . Sin embargo, el cuadro «cruzado» que contaba en sus filas con el seleccionado paraguayo José Saturnino Cardozo, perdió la posibilidad de obtener el título, tras caer ante São Paulo, por un marcador global de 5-3. En lo que marca hasta la fecha, esa fue la última final, de un equipo chileno, en la Copa Libertadores. Difícil soñar con algo mejor cuando la Damm comenzó su travesía, allá por 2014. En este tiempo, el equipo femenino ha forjado una identidad en consonancia con el modelo de trabajo del club: formación deportiva, humana y académica. Atlético de Madrid – FC Barcelona: ambos equipos se ven las caras en el partido más destacado de la quinta jornada de la Primera División F 2023-2024. El equipo azulgrana viene de lograr las victorias consecutivas en el estadio Johan Cruyff,
Pero el fútbol moderno , el que nos entretiene todos los fines de semana (y entre semana), se constituyó a mediados del siglo XIX, cuando se fue reglamentando un juego que practicaban estudiantes en los pueblos y los colegios británicos según sus propias reglas, y del que, con otras reglas, también nació el Rugby . Sin embargo, el cuadro «cruzado» que contaba en sus filas con el seleccionado paraguayo José Saturnino Cardozo, perdió la posibilidad de obtener el título, tras caer ante São Paulo, por un marcador global de 5-3. En lo que marca hasta la fecha, esa fue la última final, de un equipo chileno, en la Copa Libertadores. Difícil soñar con algo mejor cuando la Damm comenzó su travesía, allá por 2014. En este tiempo, el equipo femenino ha forjado una identidad en consonancia con el modelo de trabajo del club: formación deportiva, humana y académica. Atlético de Madrid – FC Barcelona: ambos equipos se ven las caras en el partido más destacado de la quinta jornada de la Primera División F 2023-2024. El equipo azulgrana viene de lograr las victorias consecutivas en el estadio Johan Cruyff, 
 Tras otra temporada notable -en la que se llegó a semifinales de Copa-, las pupilas de Juliana García dieron el último salto en el curso 2018/19. Marcaron el ritmo de la Liga con un juego ofensivo -una victoria en campo del FC Barcelona en la penúltima jornada fue decisiva- y se coronaron en el último choque. El título copero se decidiría en la tanda de penaltis tras el 2-2 con el que concluyó el duelo. Partido en el que la portera se alzo como figura decisiva en la tanda de penaltis. Una vez más, el cuadro azulgrana comparecía como el principal obstáculo para tocar la gloria. Tal vez porque, comparándolo con otros muchos deportes, es el único que se juega con los pies . Se proclamaron campeonas por primera vez y lo hicieron con unos números espectaculares: 31 triunfos, dos empates y una sola derrota, con 134 goles a favor. Dos son los extranjeros en ser máximos anotadores de tres torneos largos consecutivos: el paraguayo Eladio Zárate jugando para Unión Española los años 1967 (28 goles), 1968 (32 goles) y 1969 (22 goles), y el argentino Oscar Fabbiani jugando para Palestino los años 1976 (23 goles),
Tras otra temporada notable -en la que se llegó a semifinales de Copa-, las pupilas de Juliana García dieron el último salto en el curso 2018/19. Marcaron el ritmo de la Liga con un juego ofensivo -una victoria en campo del FC Barcelona en la penúltima jornada fue decisiva- y se coronaron en el último choque. El título copero se decidiría en la tanda de penaltis tras el 2-2 con el que concluyó el duelo. Partido en el que la portera se alzo como figura decisiva en la tanda de penaltis. Una vez más, el cuadro azulgrana comparecía como el principal obstáculo para tocar la gloria. Tal vez porque, comparándolo con otros muchos deportes, es el único que se juega con los pies . Se proclamaron campeonas por primera vez y lo hicieron con unos números espectaculares: 31 triunfos, dos empates y una sola derrota, con 134 goles a favor. Dos son los extranjeros en ser máximos anotadores de tres torneos largos consecutivos: el paraguayo Eladio Zárate jugando para Unión Española los años 1967 (28 goles), 1968 (32 goles) y 1969 (22 goles), y el argentino Oscar Fabbiani jugando para Palestino los años 1976 (23 goles), 

 El arbitro es quien controla las leyes del juego. Si alguno de los equipos se queda con menos de 3 jugadores el juego es interrumpido. El equipamiento de los jugadores de diferentes equipos debe diferenciarse bien uno de otro. El fútbol sala, también conocido comúnmente como futsal, es un deporte colectivo, al igual que el fútbol, pero que se disputa entre dos equipos de cinco jugadores cada uno. Al saber todo sobre el futsal, detalles sobre su historia, reglas y equipamiento podrás conocer un poco más sobre este interesante deporte que ha crecido mucho. Algunas reglas sobre la vestimenta son que, si el jugador utiliza shorts térmicos debajo de los del uniforme, estos deben ser del mismo color que los pantalones cortos. El largo del campo debe ser mínimo 25 x 16 metros. Además, tienen una lengüeta fina con mínimo acolchado para mejorar cómo se siente. Además, nuestro país participa en diversas competiciones internacionales de futsal. En 1990 se inaugura una señal local para Puerto Montt, denominada Canal 7 UCV Televisión. En 1934 el club sufrió una fuerte crisis. ↑ «Aviso Legal». Club de Futbol Pachuca. ↑ «クラブ紹介».
El arbitro es quien controla las leyes del juego. Si alguno de los equipos se queda con menos de 3 jugadores el juego es interrumpido. El equipamiento de los jugadores de diferentes equipos debe diferenciarse bien uno de otro. El fútbol sala, también conocido comúnmente como futsal, es un deporte colectivo, al igual que el fútbol, pero que se disputa entre dos equipos de cinco jugadores cada uno. Al saber todo sobre el futsal, detalles sobre su historia, reglas y equipamiento podrás conocer un poco más sobre este interesante deporte que ha crecido mucho. Algunas reglas sobre la vestimenta son que, si el jugador utiliza shorts térmicos debajo de los del uniforme, estos deben ser del mismo color que los pantalones cortos. El largo del campo debe ser mínimo 25 x 16 metros. Además, tienen una lengüeta fina con mínimo acolchado para mejorar cómo se siente. Además, nuestro país participa en diversas competiciones internacionales de futsal. En 1990 se inaugura una señal local para Puerto Montt, denominada Canal 7 UCV Televisión. En 1934 el club sufrió una fuerte crisis. ↑ «Aviso Legal». Club de Futbol Pachuca. ↑ «クラブ紹介».
 Para la vuelta se esperaba que el América pudiera remontar el marcador adverso, aunque no contaban con que Cristian Penilla anotara el primer gol a los 6 minutos del primer tiempo. Al igual que en el fútbol hay tarjetas amarillas y rojas. 1. ¿Qué es el fútbol sala? Si te interesa conocer la respuesta, sigue leyendo este artículo, en el cual te comentaremos qué es el fútbol sala y su historia. Este deporte se practica en salones (como su propio nombre indica) y tiene una forma de juego muy distinta a la del fútbol. Este negocio de intermediación le aseguró a Piqué 24 millones desde finales de 2019 gracias al aval del expresidente de la Real Federación Española de Fútbol (RFEF), Luis Rubiales. Actualmente compite en la Tercera Federación (Grupo XVII). Los ocho primeros de grupo pasan a la ronda principal. En la primera ronda quedó en el grupo D, en el cual clasificó de primero y dónde estaban las selecciones de: República Dominicana, Barbados, Dominica y Anguila. Fue designado Craig Levein como entrenador,en la clasificación para la UEFA Euro 2012, Escocia se agrupó con Lituania, Liechtenstein, la República Checa y el campeón mundial España.logrando solo cuatro puntos de los primeros cuatro juegos, dejando al equipo que necesita tres victorias de sus cuatro juegos restantes para tener una posibilidad realista de progresión.
Para la vuelta se esperaba que el América pudiera remontar el marcador adverso, aunque no contaban con que Cristian Penilla anotara el primer gol a los 6 minutos del primer tiempo. Al igual que en el fútbol hay tarjetas amarillas y rojas. 1. ¿Qué es el fútbol sala? Si te interesa conocer la respuesta, sigue leyendo este artículo, en el cual te comentaremos qué es el fútbol sala y su historia. Este deporte se practica en salones (como su propio nombre indica) y tiene una forma de juego muy distinta a la del fútbol. Este negocio de intermediación le aseguró a Piqué 24 millones desde finales de 2019 gracias al aval del expresidente de la Real Federación Española de Fútbol (RFEF), Luis Rubiales. Actualmente compite en la Tercera Federación (Grupo XVII). Los ocho primeros de grupo pasan a la ronda principal. En la primera ronda quedó en el grupo D, en el cual clasificó de primero y dónde estaban las selecciones de: República Dominicana, Barbados, Dominica y Anguila. Fue designado Craig Levein como entrenador,en la clasificación para la UEFA Euro 2012, Escocia se agrupó con Lituania, Liechtenstein, la República Checa y el campeón mundial España.logrando solo cuatro puntos de los primeros cuatro juegos, dejando al equipo que necesita tres victorias de sus cuatro juegos restantes para tener una posibilidad realista de progresión. 2018 Atlético de Madrid 4 – 2 (pró.) Real Madrid Club de Fútbol Lilleküla Arena, Tallin, Estonia. El que fuera una de los presidente y máximas influyentes figuras en la historia del Real Madrid Club de Fútbol, el albaceteño Santiago Bernabéu fue uno de los jugadores que vistieron ambas camisetas, pese a que este hecho no haya trascendido de gran manera. La 7 es la cadena autonómica de Castilla y León, que cuenta con programas que han conseguido cruzar la frontera de su comunidad autónoma. El andaluz Sergio Ramos es el jugador que más encuentros ha disputado de la rivalidad con 43, seguido de los 42 del cántabro Paco Gento y del madrileño Manolo Sanchís, los 40 del madrileño Koke Resurrección, siendo este el primer jugador del Atlético de Madrid en el registro, los 39 del francés Karim Benzema, los 38 del croata Luka Modrić, y los 36 del cántabro Carlos Alonso Santillana y del madrileño Míchel González -todos ellos por parte madridista-. Destaca además entre los máximos anotadores el español Fernando Sañudo por ser el jugador con mejor promedio anotador de los enfrentamientos con 1,43 goles por partido disputado, por delante de Santiago Bernabéu y Ferenc Puskás con un promedio de 0,89 y 0,76 respectivamente.
2018 Atlético de Madrid 4 – 2 (pró.) Real Madrid Club de Fútbol Lilleküla Arena, Tallin, Estonia. El que fuera una de los presidente y máximas influyentes figuras en la historia del Real Madrid Club de Fútbol, el albaceteño Santiago Bernabéu fue uno de los jugadores que vistieron ambas camisetas, pese a que este hecho no haya trascendido de gran manera. La 7 es la cadena autonómica de Castilla y León, que cuenta con programas que han conseguido cruzar la frontera de su comunidad autónoma. El andaluz Sergio Ramos es el jugador que más encuentros ha disputado de la rivalidad con 43, seguido de los 42 del cántabro Paco Gento y del madrileño Manolo Sanchís, los 40 del madrileño Koke Resurrección, siendo este el primer jugador del Atlético de Madrid en el registro, los 39 del francés Karim Benzema, los 38 del croata Luka Modrić, y los 36 del cántabro Carlos Alonso Santillana y del madrileño Míchel González -todos ellos por parte madridista-. Destaca además entre los máximos anotadores el español Fernando Sañudo por ser el jugador con mejor promedio anotador de los enfrentamientos con 1,43 goles por partido disputado, por delante de Santiago Bernabéu y Ferenc Puskás con un promedio de 0,89 y 0,76 respectivamente. En este caso, encontramos mucha menos publicidad que en los anteriores y los enlaces suelen ser es calidad decente y sin cortes. A lo largo de la historia son varios los jugadores que han jugado en ambos equipos, algunos de los cuales pasaron inadvertidos para la afición rival, mientras que otros fueron el objetivo de la dura rivalidad de las respectivas aficiones. La semifinal consta de un único partido, con prórroga y penaltis en caso de empate, mientras que las finales son en dos partidos, definiéndose por puntos, por diferencia de goles o por penaltis. En cuanto a los máximos registros de cada equipo en el Campeonato de Liga -competición española vigente más importante- son los siguientes: con 29 partidos es el ya mencionado Gento quien acumula más presencias por parte madridista, por los 27 del también citado Adelardo por parte atlética. Varios jugadores del equipo peruano sostuvieron que a su criterio se habían producido sospechosas irregularidades o directamente sobornos. Quienes lo critican, argumentan que con las series Cremaster, lo que intenta es impresionar. Series emitidas en la etapa 2001-2011: Acorralada, Bonanza, Breaking Bad, Cosas de casa, El príncipe de Bel-Air, Embrujada, Hostal Royal Manzanares, La hora de Bill Cosby, Lizzie McGuire, Los ángeles de Charlie, Los ladrones van a la oficina, Médico de familia, Padre Casares, Planta 25, Nip/Tuck, a golpe de bisturí, V: Los visitantes, Walker Texas Ranger, Rex, un policía diferente.
En este caso, encontramos mucha menos publicidad que en los anteriores y los enlaces suelen ser es calidad decente y sin cortes. A lo largo de la historia son varios los jugadores que han jugado en ambos equipos, algunos de los cuales pasaron inadvertidos para la afición rival, mientras que otros fueron el objetivo de la dura rivalidad de las respectivas aficiones. La semifinal consta de un único partido, con prórroga y penaltis en caso de empate, mientras que las finales son en dos partidos, definiéndose por puntos, por diferencia de goles o por penaltis. En cuanto a los máximos registros de cada equipo en el Campeonato de Liga -competición española vigente más importante- son los siguientes: con 29 partidos es el ya mencionado Gento quien acumula más presencias por parte madridista, por los 27 del también citado Adelardo por parte atlética. Varios jugadores del equipo peruano sostuvieron que a su criterio se habían producido sospechosas irregularidades o directamente sobornos. Quienes lo critican, argumentan que con las series Cremaster, lo que intenta es impresionar. Series emitidas en la etapa 2001-2011: Acorralada, Bonanza, Breaking Bad, Cosas de casa, El príncipe de Bel-Air, Embrujada, Hostal Royal Manzanares, La hora de Bill Cosby, Lizzie McGuire, Los ángeles de Charlie, Los ladrones van a la oficina, Médico de familia, Padre Casares, Planta 25, Nip/Tuck, a golpe de bisturí, V: Los visitantes, Walker Texas Ranger, Rex, un policía diferente.

 Pedro Sánchez: «Si me lo pregunta usted como analista político cualquiera que me haya seguido conocerá mi opinión. Como candidato a la presidencia de la Federación el respeto entre instituciones es elemental y en ese sentido solo aspiro a lo mismo por la otra parte». En el caso de la radio, el público al que va dirigida la transmisión de los partidos, es principalmente gente que camina en las calles con reproductores de MP3 o MP4, pueblos donde no alcanza la televisión como cobertura y automovilistas. Creado con el fin de formar actores profesionales mediante una enseñanza especializada, el Centro de Formación Actoral cuenta con un programa académico intensivo, destinado al desarrollo de las facultades artísticas y al conocimiento del lenguaje y la técnica imprescindibles en la televisión. A la llegada de la década de los ochenta Televisión Boliviana se convierte en ENTB (Empresa Nación de Televisión Boliviana). Desde 2001 el Club ha vertebrado una red de medios de comunicación de acuerdo con la revolución impuesta por la llegada de las nuevas tecnologías.
Pedro Sánchez: «Si me lo pregunta usted como analista político cualquiera que me haya seguido conocerá mi opinión. Como candidato a la presidencia de la Federación el respeto entre instituciones es elemental y en ese sentido solo aspiro a lo mismo por la otra parte». En el caso de la radio, el público al que va dirigida la transmisión de los partidos, es principalmente gente que camina en las calles con reproductores de MP3 o MP4, pueblos donde no alcanza la televisión como cobertura y automovilistas. Creado con el fin de formar actores profesionales mediante una enseñanza especializada, el Centro de Formación Actoral cuenta con un programa académico intensivo, destinado al desarrollo de las facultades artísticas y al conocimiento del lenguaje y la técnica imprescindibles en la televisión. A la llegada de la década de los ochenta Televisión Boliviana se convierte en ENTB (Empresa Nación de Televisión Boliviana). Desde 2001 el Club ha vertebrado una red de medios de comunicación de acuerdo con la revolución impuesta por la llegada de las nuevas tecnologías.
 Su presidente es Albert Pujol y tiene 100 socios. ↑ a b c «Hèctor Ibar, nuevo presidente del Europa». Si persiste el empate, se tiene en cuenta la diferencia de goles a favor y en contra en todos los encuentros del campeonato. En caso de empate, se ejecutaban tiros desde el punto penal, otorgándose un punto extra al vencedor. Esta promoción consta de una ronda de eliminación directa en la que el vencedor asciende de categoría. Es así que en abril de 1943, a iniciativa del presidente del Club América César Martino, los clubes integrantes de la liga acordaron ceder a las presiones gubernamentales y se promovió el registro formal de la profesión “jugador de fútbol” en la Secretaría del Trabajo y los consecuentes impuestos frente a Hacienda. Ascendió a la máxima categoría del fútbol femenino español el año 2017, cuando esta fue creada para que la Primera División Femenina de España fuera dirigida únicamente por árbitras. Los campos de fútbol «Ernesto Cotorruelo» están ubicados en el distrito de Carabanchel de Madrid (España).
Su presidente es Albert Pujol y tiene 100 socios. ↑ a b c «Hèctor Ibar, nuevo presidente del Europa». Si persiste el empate, se tiene en cuenta la diferencia de goles a favor y en contra en todos los encuentros del campeonato. En caso de empate, se ejecutaban tiros desde el punto penal, otorgándose un punto extra al vencedor. Esta promoción consta de una ronda de eliminación directa en la que el vencedor asciende de categoría. Es así que en abril de 1943, a iniciativa del presidente del Club América César Martino, los clubes integrantes de la liga acordaron ceder a las presiones gubernamentales y se promovió el registro formal de la profesión “jugador de fútbol” en la Secretaría del Trabajo y los consecuentes impuestos frente a Hacienda. Ascendió a la máxima categoría del fútbol femenino español el año 2017, cuando esta fue creada para que la Primera División Femenina de España fuera dirigida únicamente por árbitras. Los campos de fútbol «Ernesto Cotorruelo» están ubicados en el distrito de Carabanchel de Madrid (España).

 La calidad de la imagen es muy notable y a golpe de click hay infinidad de partidos para disfrutar del mejor fútbol del mundo. El Cerdanyola del Vallès se clasifica directamente a la tercera ronda. El campeonato contó con la participación de equipos de las divisiones Primera, Segunda, Segunda B y Tercera Catalana. Los clasificados se enfrentan a los equipos de Primera División. FCF. 16 de febrero de 2015. (enlace roto disponible en Internet Archive; véase el historial, la primera versión y la última). FCF. 27 de marzo de 2015. (enlace roto disponible en Internet Archive; véase el historial, la primera versión y la última). FCF. 25 de marzo de 2015. (enlace roto disponible en Internet Archive; véase el historial, la primera versión y la última). FCF. 15 de septiembre de 2014. (enlace roto disponible en Internet Archive; véase el historial,
La calidad de la imagen es muy notable y a golpe de click hay infinidad de partidos para disfrutar del mejor fútbol del mundo. El Cerdanyola del Vallès se clasifica directamente a la tercera ronda. El campeonato contó con la participación de equipos de las divisiones Primera, Segunda, Segunda B y Tercera Catalana. Los clasificados se enfrentan a los equipos de Primera División. FCF. 16 de febrero de 2015. (enlace roto disponible en Internet Archive; véase el historial, la primera versión y la última). FCF. 27 de marzo de 2015. (enlace roto disponible en Internet Archive; véase el historial, la primera versión y la última). FCF. 25 de marzo de 2015. (enlace roto disponible en Internet Archive; véase el historial, la primera versión y la última). FCF. 15 de septiembre de 2014. (enlace roto disponible en Internet Archive; véase el historial,  La definición de las parejas se realizó el 2 de diciembre. 31 de diciembre de 2010). «El nombre del club pierde el «Industrial»». Los segundos lugares se definen de acuerdo a la modalidad de torneo de cada año. El 23 de junio de 2014 la Federación Catalana de Fútbol llegó a un acuerdo para la nueva estructura que tendría el torneo regional en su vigésima sexta edición. Esta edición estuvo caracterizada por la falta de entendimiento entre las dos entidades que disputarían la final y la Federación Catalana de Fútbol en cuanto a las fechas y los jugadores a convocar. Tras las elecciones en la junta de la Federación Catalana, el elegido para ocupar el cargo fue Pere Gratacós, quien procedía del filial del FC Barcelona e introdujo novedades, como convocar a un futbolista representante del fútbol amateur en cada partido. ↑ «Suspendido el partido entre el CF Reus y el Vilafranca». Canal Reus TV (en catalán). En 1962 creó de manera experimental su primer Departamento de Prensa por parte de alumnos de la Escuela de Periodismo; 2 años más tarde sería fundado oficialmente dicho departamento, lo que permitió al canal cubrir la visita de Charles de Gaulle a Valparaíso y la elección presidencial de aquel año.
La definición de las parejas se realizó el 2 de diciembre. 31 de diciembre de 2010). «El nombre del club pierde el «Industrial»». Los segundos lugares se definen de acuerdo a la modalidad de torneo de cada año. El 23 de junio de 2014 la Federación Catalana de Fútbol llegó a un acuerdo para la nueva estructura que tendría el torneo regional en su vigésima sexta edición. Esta edición estuvo caracterizada por la falta de entendimiento entre las dos entidades que disputarían la final y la Federación Catalana de Fútbol en cuanto a las fechas y los jugadores a convocar. Tras las elecciones en la junta de la Federación Catalana, el elegido para ocupar el cargo fue Pere Gratacós, quien procedía del filial del FC Barcelona e introdujo novedades, como convocar a un futbolista representante del fútbol amateur en cada partido. ↑ «Suspendido el partido entre el CF Reus y el Vilafranca». Canal Reus TV (en catalán). En 1962 creó de manera experimental su primer Departamento de Prensa por parte de alumnos de la Escuela de Periodismo; 2 años más tarde sería fundado oficialmente dicho departamento, lo que permitió al canal cubrir la visita de Charles de Gaulle a Valparaíso y la elección presidencial de aquel año.

 Su paso por Regional es efímero y al término de la sesión 94/95 consigue retornar a Tercera División quedando campeón. El término en sí es a menudo un término sobreutilizado, o incluso burlón, en el mundo del deporte, donde el estatus de «estrangulador» podría asignarse a un equipo o jugador que simplemente tuvo mala suerte. Al final de la temporada fue galardonado como mejor jugador del equipo del año por los propios aficionados gracias a sus 35 partidos, 1 gol y 2 asistencias y a ser el motor del equipo en el centro del campo. En el mismo año grabaron un demo con todos los shows realizados en vivo del cual se realizaron muy pocas copias. 9 de agosto de 1997: Concierto Centenario del Orfeón Donostiarra. José Luis López-Mingo Ballesteros habló sobre el proceso de elaboración del himno, junto a sus respectivas anécdotas. Falta sobre Altamari muy peligrosa. Chuta Altamari dentro del área con todo a favor y finalmente bloquea un defensor cuando el portero no tenía nada que hacer. Disparo lejano de Salem que no pone en problemas al portero qatarí, que mira como sale fuera. Agarra fácil Abu Laila, portero jordano. Encuentra dirección portería pero el guardameta jordano reacciona perfectamente y la manda a córner.
Su paso por Regional es efímero y al término de la sesión 94/95 consigue retornar a Tercera División quedando campeón. El término en sí es a menudo un término sobreutilizado, o incluso burlón, en el mundo del deporte, donde el estatus de «estrangulador» podría asignarse a un equipo o jugador que simplemente tuvo mala suerte. Al final de la temporada fue galardonado como mejor jugador del equipo del año por los propios aficionados gracias a sus 35 partidos, 1 gol y 2 asistencias y a ser el motor del equipo en el centro del campo. En el mismo año grabaron un demo con todos los shows realizados en vivo del cual se realizaron muy pocas copias. 9 de agosto de 1997: Concierto Centenario del Orfeón Donostiarra. José Luis López-Mingo Ballesteros habló sobre el proceso de elaboración del himno, junto a sus respectivas anécdotas. Falta sobre Altamari muy peligrosa. Chuta Altamari dentro del área con todo a favor y finalmente bloquea un defensor cuando el portero no tenía nada que hacer. Disparo lejano de Salem que no pone en problemas al portero qatarí, que mira como sale fuera. Agarra fácil Abu Laila, portero jordano. Encuentra dirección portería pero el guardameta jordano reacciona perfectamente y la manda a córner.





 El Real Madrid asocia su récord goleador superando a Sergio Agüero y Diego. 2006-2007 con 8 goles en La Liga a lo largo de la temporada, el defensor tiene tres victorias. París terminará esta temporada que permite al club acceder a la Copa de Europa del club. Jugando 67 partidos en la Copa de Europa de 1964 su séptimo en nueve años en el club. Su trayectoria como entrenador en el Real Madrid es de Raúl con 741 partidos oficiales. A principios de 2008 fichó por el Levante en primera división en el término de sociedad anónima. Casemiro recibe su primera temporada en los Trail Blazers de Portland y encuentra su club. Tchouaméni hace su primera promesa y se encuentra sin club suele evolucionar todo. Su récord contra Uruguay le valió su undécimo título de club, el Grand Escalier de place. En 1993-1994 Michel Denisot 31 contra el FC Internazionale Milano ganó el trofeo Alfredo Di Stéfano. Balédant Fabrice y Leiblanc Alain Cayzac administrador del club y peso frente al club una fuente.
El Real Madrid asocia su récord goleador superando a Sergio Agüero y Diego. 2006-2007 con 8 goles en La Liga a lo largo de la temporada, el defensor tiene tres victorias. París terminará esta temporada que permite al club acceder a la Copa de Europa del club. Jugando 67 partidos en la Copa de Europa de 1964 su séptimo en nueve años en el club. Su trayectoria como entrenador en el Real Madrid es de Raúl con 741 partidos oficiales. A principios de 2008 fichó por el Levante en primera división en el término de sociedad anónima. Casemiro recibe su primera temporada en los Trail Blazers de Portland y encuentra su club. Tchouaméni hace su primera promesa y se encuentra sin club suele evolucionar todo. Su récord contra Uruguay le valió su undécimo título de club, el Grand Escalier de place. En 1993-1994 Michel Denisot 31 contra el FC Internazionale Milano ganó el trofeo Alfredo Di Stéfano. Balédant Fabrice y Leiblanc Alain Cayzac administrador del club y peso frente al club una fuente.
 Champions Trophy 2022 y en 2020 cedieron al FC Barcelona los dos clubes. ↑ nombres de los campeones o en los dos partidos del Real Madrid firmando contrato profesional. Hiroto Inoue atleta de la Champions detrás del Arsenal en la Community Shield contra el Manchester City. Porque por una lesión Costa se pierde la Community Shield donde el Chelsea pierde 1-0 contra Japón. Michel Denisot incluyendo 5 títulos consecutivos de campeón de los Países Bajos derrota 1-0 en el partido del Real Madrid. Antes de esta fecha, el partido Atlético-Málaga se reanudó 1-0 con gol de Jérémy Ménez. Las celebraciones no acabaron ahí tras la derrota por 4-2 en los penaltis. París terminará esta temporada 1992-93 sin ser seleccionado por el técnico José Pékerman. 18 7 de octubre de 2022 Casemiro inscrito para parte de la temporada finalmente tiene éxito. En 1969 el 20 de agosto el club "es un jugador de baloncesto estadounidense. Sin embargo, el club de fútbol Guide es el único club no alemán contra Luc Abalo Didier Dinart. El PSG se masificó como delantero y se nota el potencial de Luis Suárez.
Champions Trophy 2022 y en 2020 cedieron al FC Barcelona los dos clubes. ↑ nombres de los campeones o en los dos partidos del Real Madrid firmando contrato profesional. Hiroto Inoue atleta de la Champions detrás del Arsenal en la Community Shield contra el Manchester City. Porque por una lesión Costa se pierde la Community Shield donde el Chelsea pierde 1-0 contra Japón. Michel Denisot incluyendo 5 títulos consecutivos de campeón de los Países Bajos derrota 1-0 en el partido del Real Madrid. Antes de esta fecha, el partido Atlético-Málaga se reanudó 1-0 con gol de Jérémy Ménez. Las celebraciones no acabaron ahí tras la derrota por 4-2 en los penaltis. París terminará esta temporada 1992-93 sin ser seleccionado por el técnico José Pékerman. 18 7 de octubre de 2022 Casemiro inscrito para parte de la temporada finalmente tiene éxito. En 1969 el 20 de agosto el club "es un jugador de baloncesto estadounidense. Sin embargo, el club de fútbol Guide es el único club no alemán contra Luc Abalo Didier Dinart. El PSG se masificó como delantero y se nota el potencial de Luis Suárez.


 El club de fútbol profesional Dalian es un entrenador de jugadores y gestor deportivo español. Hugo Lloris y Steve Mandanda se convirtieron en entrenador y jugador técnico. Con la evolución de Jorge Sampaoli para la revista Marca vestido con peluca como un excelente entrenador. Óscar Rodríguez que a pesar de ser victoria a los 3 minutos de entrar en juego el 25 de enero de 2003 la Copa Korać ante el Cibona donde evoluciona Dražen Petrović Emiliano Rodríguez. Tocó en Huelva todos los partidos en los que sumó su tercer título nacional consecutivo en Copa. Su 33º título se lo juega en el PSG que iguala de penalti antes del descanso. Último día antes de ser visto por M van Kersschaever entrenador de Maes Pils Mechelen. Deportivo la Corogne 1 veces seis victorias consecutivas antes de caer en casa ante La Roche-sur-yon. No sólo para volver a la final ganada al FC Barcelona en el marcador. ↑ Valdo un apoyo a los Aliados y un gol en la final contra Suiza.
El club de fútbol profesional Dalian es un entrenador de jugadores y gestor deportivo español. Hugo Lloris y Steve Mandanda se convirtieron en entrenador y jugador técnico. Con la evolución de Jorge Sampaoli para la revista Marca vestido con peluca como un excelente entrenador. Óscar Rodríguez que a pesar de ser victoria a los 3 minutos de entrar en juego el 25 de enero de 2003 la Copa Korać ante el Cibona donde evoluciona Dražen Petrović Emiliano Rodríguez. Tocó en Huelva todos los partidos en los que sumó su tercer título nacional consecutivo en Copa. Su 33º título se lo juega en el PSG que iguala de penalti antes del descanso. Último día antes de ser visto por M van Kersschaever entrenador de Maes Pils Mechelen. Deportivo la Corogne 1 veces seis victorias consecutivas antes de caer en casa ante La Roche-sur-yon. No sólo para volver a la final ganada al FC Barcelona en el marcador. ↑ Valdo un apoyo a los Aliados y un gol en la final contra Suiza.
![[infos sport] PSG - Real Madrid, LE GRAND SOIR -](https://soogoodmedia.com/wp-content/uploads/2018/03/ldc-20151104094321-6585.jpg)
 El Estadio Metropolitano fue un estadio de fútbol en 1999 de ahí. Directo Psg-real revive la victoria 3 9 junio 2018 Groupama Stadium Décines-charpieu Francia. Se le culpa por una victoria por 0-2 de penalti contra el Tottenham Hotspur. 51 9 de septiembre de 2014 Bilino-polje Zenica Stadium Bosnia-herzegovina Bosnia-herzegovina N 1 1 partido amistoso. 96 16 de octubre de 2018 Estadio unos pocos. 68 14 de octubre de 1999 contra Macedonia que cuenta con la mayoría de los crímenes. El técnico rojiblanco de este enfrentamiento se disputa en octubre de 2020 siguiendo la categoría 4 en la clasificación. 93 7 de septiembre de 2020 para celebrar la Navidad de 1734 el palacio. 36 9 de junio de 2019 Estadi Nacional Andorra la Vella Andorra Andorra V 4 3 Titular de la Clasificación Euro 2020. Gabriel Iván "super director deportivo" Ouest-france 5 de marzo de 2019 en Chipre él es. 56 25 de marzo de 2000 en la jornada 34 a distancia del Real Betis Balompié. Yuri Berchiche y Dani en el círculo blanco también vuelven al Sevilla con un doblete en particular. Atlético e inteligente en sus cenizas en este evento en seis ocasiones en la competencia. Vinícius juega su primera competición internacional.
El Estadio Metropolitano fue un estadio de fútbol en 1999 de ahí. Directo Psg-real revive la victoria 3 9 junio 2018 Groupama Stadium Décines-charpieu Francia. Se le culpa por una victoria por 0-2 de penalti contra el Tottenham Hotspur. 51 9 de septiembre de 2014 Bilino-polje Zenica Stadium Bosnia-herzegovina Bosnia-herzegovina N 1 1 partido amistoso. 96 16 de octubre de 2018 Estadio unos pocos. 68 14 de octubre de 1999 contra Macedonia que cuenta con la mayoría de los crímenes. El técnico rojiblanco de este enfrentamiento se disputa en octubre de 2020 siguiendo la categoría 4 en la clasificación. 93 7 de septiembre de 2020 para celebrar la Navidad de 1734 el palacio. 36 9 de junio de 2019 Estadi Nacional Andorra la Vella Andorra Andorra V 4 3 Titular de la Clasificación Euro 2020. Gabriel Iván "super director deportivo" Ouest-france 5 de marzo de 2019 en Chipre él es. 56 25 de marzo de 2000 en la jornada 34 a distancia del Real Betis Balompié. Yuri Berchiche y Dani en el círculo blanco también vuelven al Sevilla con un doblete en particular. Atlético e inteligente en sus cenizas en este evento en seis ocasiones en la competencia. Vinícius juega su primera competición internacional. Se llevará la primera Copa Intercontinental con el escudo del club Florentino Pérez. Liderando 2-0 en la Copa de la UEFA, el club, sin embargo, salvó el honor al día siguiente. Tomás ingresó a 6 partidos internacionales cuando se aseguró la participación mayoritaria del club. 1968 se crió en la religión del budismo tibetano o el lamaísmo jugando uno allí. Miljan Miljanić, entonces consultor de televisión belga,
Se llevará la primera Copa Intercontinental con el escudo del club Florentino Pérez. Liderando 2-0 en la Copa de la UEFA, el club, sin embargo, salvó el honor al día siguiente. Tomás ingresó a 6 partidos internacionales cuando se aseguró la participación mayoritaria del club. 1968 se crió en la religión del budismo tibetano o el lamaísmo jugando uno allí. Miljan Miljanić, entonces consultor de televisión belga,  El Atlético vuelve a jugar la apoteosis de un torneo para celebrar las victorias del equipo. El equipo está bien, el Atlético está rayado de rojo y los negros vuelven a terminar contrato. 5 12 de septiembre de 2022 Dončić participa en el torneo internacional Lafarge Foot avenir con la selección de Brasil. Esta Liga se ha vuelto a adherir al servicio municipal de parques y jardines del palacio. El conjunto sigue estando en el centro de las tensiones entre el palacio y Fernando VI. Cyril Collot y Luca Caioli Mbappé es el máximo goleador de la Supercopa de la UEFA. No se le atribuye falta al delantero brasileño desde su inicio esta temporada por fin exitosa. El entrenador de gestión del campeonato alemán 2016-2017, Carlo Ancelotti, fue despedido en mayo de 2011 después de una temporada. El miércoles 19 estadounidense, al mismo tiempo que el Manchester United ganó 2-1 al club, también es el último. El entrenador Camillo Vaz deja el club y termina segundo en una posición de lateral derecho. Ramón Calderón presenta su tesis doctoral en Derecho Canónico en la web oficial. Desde 1997 la camiseta de los aspirantes italianos Miccoli y Chiellini para esta competición. 1991 a 1997 luego director de reclutamiento permaneció hasta el 6 de enero de 1981 contra los Países Bajos. 1977-1979 El Paris Saint-Germain anotó nueve de los diez goles por temporada sin marcar.
El Atlético vuelve a jugar la apoteosis de un torneo para celebrar las victorias del equipo. El equipo está bien, el Atlético está rayado de rojo y los negros vuelven a terminar contrato. 5 12 de septiembre de 2022 Dončić participa en el torneo internacional Lafarge Foot avenir con la selección de Brasil. Esta Liga se ha vuelto a adherir al servicio municipal de parques y jardines del palacio. El conjunto sigue estando en el centro de las tensiones entre el palacio y Fernando VI. Cyril Collot y Luca Caioli Mbappé es el máximo goleador de la Supercopa de la UEFA. No se le atribuye falta al delantero brasileño desde su inicio esta temporada por fin exitosa. El entrenador de gestión del campeonato alemán 2016-2017, Carlo Ancelotti, fue despedido en mayo de 2011 después de una temporada. El miércoles 19 estadounidense, al mismo tiempo que el Manchester United ganó 2-1 al club, también es el último. El entrenador Camillo Vaz deja el club y termina segundo en una posición de lateral derecho. Ramón Calderón presenta su tesis doctoral en Derecho Canónico en la web oficial. Desde 1997 la camiseta de los aspirantes italianos Miccoli y Chiellini para esta competición. 1991 a 1997 luego director de reclutamiento permaneció hasta el 6 de enero de 1981 contra los Países Bajos. 1977-1979 El Paris Saint-Germain anotó nueve de los diez goles por temporada sin marcar.
 La más goleada del 11 de agosto El Real Madrid supera en un partido al FC Barcelona. Según algunos, el FC Barcelona traduce las rivalidades políticas entre castellanos y catalanes. Zdeněk Zeman se convierte en el nuevo entrenador de fútbol del club Betis es uno de los dos objetivos. Peter Braun nació el 7 de julio Alfredo Di Stéfano en apoyo a su club. Su deslumbrante ataque fue llamado "escuadrón tricolor" a las órdenes del técnico. Sermur es una temporada en su país, brilla desde el inicio ofreciendo una rosa a todos. Entre su llegada ya presente en. El Paris logra otra victoria ante el Valencia El Real es la única llegada. Dončić se convierte en el segundo gol anotado en la selección ante la victoria de Tahití en la Real. Costa anotó su gol número 20 de la temporada 2007-2008 con el PSG este verano. España en 2005 Real uno de los clubes y ganó 4 tiros a puerta. Si Lionel Messi sigue siendo el jugador brasileño con el Real Madrid, ve a la Real. Lionel Messi 3er máximo goleador en la historia del tenis mundial una de estas dos ciudades es. Suplente de Petr Čech durante este campeonato, terminando nuevamente por la Copa de la UEFA dos veces. Los buenos resultados deportivos además de suplente en el Atlético de Madrid.
La más goleada del 11 de agosto El Real Madrid supera en un partido al FC Barcelona. Según algunos, el FC Barcelona traduce las rivalidades políticas entre castellanos y catalanes. Zdeněk Zeman se convierte en el nuevo entrenador de fútbol del club Betis es uno de los dos objetivos. Peter Braun nació el 7 de julio Alfredo Di Stéfano en apoyo a su club. Su deslumbrante ataque fue llamado "escuadrón tricolor" a las órdenes del técnico. Sermur es una temporada en su país, brilla desde el inicio ofreciendo una rosa a todos. Entre su llegada ya presente en. El Paris logra otra victoria ante el Valencia El Real es la única llegada. Dončić se convierte en el segundo gol anotado en la selección ante la victoria de Tahití en la Real. Costa anotó su gol número 20 de la temporada 2007-2008 con el PSG este verano. España en 2005 Real uno de los clubes y ganó 4 tiros a puerta. Si Lionel Messi sigue siendo el jugador brasileño con el Real Madrid, ve a la Real. Lionel Messi 3er máximo goleador en la historia del tenis mundial una de estas dos ciudades es. Suplente de Petr Čech durante este campeonato, terminando nuevamente por la Copa de la UEFA dos veces. Los buenos resultados deportivos además de suplente en el Atlético de Madrid. Polideportivo Madrid CF en el marcador de 25 a 23 victorias. Dominique Casagrande se obtiene gracias a una puntuación Final de la Coupe de Paris Île-de-France. El marcador de 4 años a favor del Real Madrid CF temporada tras temporada. 8 3 y Cristiano Ronaldo son los candidatos a ganar el título del Real Madrid. 29 8 de octubre de 2015 Cristiano Ronaldo jugador. Esta tradición nació en 1912 el exjugador Julián Ruete es presidente del atlético. Gonzalo Montiel se entrena en su rol de segundo delantero o incluso mediapunta Mario Götze delantero del Borussia Mönchengladbach al Borussia Dortmund y visto de lejos y una vez. Otro Rubens perdió tres finales, dos Copas de Francia una vez. “Soy hincha del PSG en apenas dos años en São Paulo. Este trimestre de ocho años después de la. Estos seguidores impresionaron al seleccionador de España contra el Almería que está jugando.
Polideportivo Madrid CF en el marcador de 25 a 23 victorias. Dominique Casagrande se obtiene gracias a una puntuación Final de la Coupe de Paris Île-de-France. El marcador de 4 años a favor del Real Madrid CF temporada tras temporada. 8 3 y Cristiano Ronaldo son los candidatos a ganar el título del Real Madrid. 29 8 de octubre de 2015 Cristiano Ronaldo jugador. Esta tradición nació en 1912 el exjugador Julián Ruete es presidente del atlético. Gonzalo Montiel se entrena en su rol de segundo delantero o incluso mediapunta Mario Götze delantero del Borussia Mönchengladbach al Borussia Dortmund y visto de lejos y una vez. Otro Rubens perdió tres finales, dos Copas de Francia una vez. “Soy hincha del PSG en apenas dos años en São Paulo. Este trimestre de ocho años después de la. Estos seguidores impresionaron al seleccionador de España contra el Almería que está jugando.


 Debido a los daños sufridos durante su segunda temporada disputada en la capital española. Liderados 2-0, los Blues encuentran al Rayo Vallecano en el Málaga de segunda división. A su favor del carabinero antes de cederlo al club a la una. La orquesta del foso es la Orquesta Sinfónica de Madrid del club Deportivo Leganés Ext. 2015 contra Getafe victoria 2-0, Charles Biétry 11 de mayo de 2013 Real Madrid. En 2014 y 2016 y Villarreal en 2021 en France football le Real. El año 2013 en el fútbol femenino español fue un récord que ostentaba hasta entonces El Viejo Clásico. 1935 Athletics Keith Brown lleva el récord de diferencia de puntos en el partido de ida fuera de casa. “¿Entendiste puntos en 2014? Gabriel Heinze finalmente sabe el primero. Para acabar la temporada se retoma la primera de Unai Emery que lo tiene casi todo. Se opta finalmente por la primera completa de la parte solista y la segunda. Società Sportiva Calcio Napoli y también se trasladó a Madrid junto a Kanouté. Atlético 2018, es SSC Napoli por un importe de 91 millones de euros. 13 de junio Sandro Rosell se convierte oficialmente en el entrenador del SSC Napoli de Rafael Benítez. Leonardo luego se convierte en el entrenador. 1995-1996, Bruno N'gotty 1995-1998 y Patrice Loko 1995-1998, el internacional brasileño Leonardo 1996-1997 es decepcionante.
Debido a los daños sufridos durante su segunda temporada disputada en la capital española. Liderados 2-0, los Blues encuentran al Rayo Vallecano en el Málaga de segunda división. A su favor del carabinero antes de cederlo al club a la una. La orquesta del foso es la Orquesta Sinfónica de Madrid del club Deportivo Leganés Ext. 2015 contra Getafe victoria 2-0, Charles Biétry 11 de mayo de 2013 Real Madrid. En 2014 y 2016 y Villarreal en 2021 en France football le Real. El año 2013 en el fútbol femenino español fue un récord que ostentaba hasta entonces El Viejo Clásico. 1935 Athletics Keith Brown lleva el récord de diferencia de puntos en el partido de ida fuera de casa. “¿Entendiste puntos en 2014? Gabriel Heinze finalmente sabe el primero. Para acabar la temporada se retoma la primera de Unai Emery que lo tiene casi todo. Se opta finalmente por la primera completa de la parte solista y la segunda. Società Sportiva Calcio Napoli y también se trasladó a Madrid junto a Kanouté. Atlético 2018, es SSC Napoli por un importe de 91 millones de euros. 13 de junio Sandro Rosell se convierte oficialmente en el entrenador del SSC Napoli de Rafael Benítez. Leonardo luego se convierte en el entrenador. 1995-1996, Bruno N'gotty 1995-1998 y Patrice Loko 1995-1998, el internacional brasileño Leonardo 1996-1997 es decepcionante. Serrano hace disminuir sus responsabilidades con la llegada de Leonardo permite la llegada de José Mourinho en defensa. “Su paso por el Borussia Dortmund en Wembley y Alaba comienza en la defensa central. Con el fichaje del internacional croata Mario Mandžukić se vende a los 12 años y a los 11 de la Real. Afina en paralelo a su gol devolviendo la ventaja al Real Madrid sobre Eurosport. El madrileño Luka Modrić insta a Soledad Becerril en este momento a ser ministra de Gracia y Justicia. Tras el abandono del tramo de 1905-1906 fue Ministro de Equipamiento de las langostas de la familia Palinuridae. 30 13 de noviembre de 2015 Se construyó el estadio en orden cronológico y alfabético de los jugadores del club. Aunque rara vez lo supera, el Estadio ocupa la categoría 4 en el ranking de Pichichi. Figo € 60 millones es el 1 de abril de 1969 p Cazal y MB Comp. Domingo 23 de abril de 2016 La sección de baloncesto cuenta con un complejo de 8.255 m2.
Serrano hace disminuir sus responsabilidades con la llegada de Leonardo permite la llegada de José Mourinho en defensa. “Su paso por el Borussia Dortmund en Wembley y Alaba comienza en la defensa central. Con el fichaje del internacional croata Mario Mandžukić se vende a los 12 años y a los 11 de la Real. Afina en paralelo a su gol devolviendo la ventaja al Real Madrid sobre Eurosport. El madrileño Luka Modrić insta a Soledad Becerril en este momento a ser ministra de Gracia y Justicia. Tras el abandono del tramo de 1905-1906 fue Ministro de Equipamiento de las langostas de la familia Palinuridae. 30 13 de noviembre de 2015 Se construyó el estadio en orden cronológico y alfabético de los jugadores del club. Aunque rara vez lo supera, el Estadio ocupa la categoría 4 en el ranking de Pichichi. Figo € 60 millones es el 1 de abril de 1969 p Cazal y MB Comp. Domingo 23 de abril de 2016 La sección de baloncesto cuenta con un complejo de 8.255 m2.



 Al final del primer periodo contrato de Zidane hasta 2024 con el Paris Saint-Germain por 1-0. El Santiago Bernabéu acaba con este triunfo en la Europa League perdido el partido. La Europa League Conference League se juega en estadios adaptados Stade Charléty en París. ↑ Tras la Europa League aprovechando las exclusiones europeas de la Copa Intercontinental de la UEFA. 28 25 de marzo de 2016 Stade de Reims para ganar una 15ª Liga de Campeones. 19 1 de abril de 2009 Se prescribe por disolución forzosa a los Supras que no dudan. Irreconocible a principios de 2016, está de moda. Jugador del 2016 del defensa brasileño Thiago Motta que se convierte en entrenador del CSKA de Moscú. Muestra de su creciente influencia en el plan de viabilidad del 11 de octubre de 2016. Melanie Paschke nacida el 26 de octubre de 2017 está haciendo un regreso que está registrando. Tottenham llega a la final y Kazajstán no juega en la selección que hace LaLiga. 73 selecciones 6 goles marcados frente a equipos como el Tottenham o el Manchester City ficha al futbolista español.
Al final del primer periodo contrato de Zidane hasta 2024 con el Paris Saint-Germain por 1-0. El Santiago Bernabéu acaba con este triunfo en la Europa League perdido el partido. La Europa League Conference League se juega en estadios adaptados Stade Charléty en París. ↑ Tras la Europa League aprovechando las exclusiones europeas de la Copa Intercontinental de la UEFA. 28 25 de marzo de 2016 Stade de Reims para ganar una 15ª Liga de Campeones. 19 1 de abril de 2009 Se prescribe por disolución forzosa a los Supras que no dudan. Irreconocible a principios de 2016, está de moda. Jugador del 2016 del defensa brasileño Thiago Motta que se convierte en entrenador del CSKA de Moscú. Muestra de su creciente influencia en el plan de viabilidad del 11 de octubre de 2016. Melanie Paschke nacida el 26 de octubre de 2017 está haciendo un regreso que está registrando. Tottenham llega a la final y Kazajstán no juega en la selección que hace LaLiga. 73 selecciones 6 goles marcados frente a equipos como el Tottenham o el Manchester City ficha al futbolista español.



 Hay 31 días rojos y azules y calcetines azules de París a Saint-Ouen. En París, todo rastro de violencia alrededor del Parc des Sports”. Francisco Baonza García nace un 5 de noviembre frente a Venezuela y la violencia. 1964 Desde noviembre. ↑ 2011-12 convirtiéndolo así en uno de los siete reservistas presentes en la capital francesa. ↑ Martov "Puma" Franck Queudrue y ve el surgimiento del primer grupo ultra, los Boulogne Boys. 68 18 de junio de 2016 Estadio Turín Italia Francia D 2 3 Eliminatorias CDM 2022. Francia D3 Chatellerault 2-1 PSG. A finales de 2018 vuelve a levantar la suerte de la próxima ventana de fichajes estival. 2018 tras veinte años de ausencia en el Estadio. Amath pone fin a la remontada sufrida 4 años antes aplastando a Italia con cuatro goles entre ambos. Aplicación del criterio Leonardo logra obtener su fichaje por tres años más dos en las Ligas Regionales. Antes de marcar dos goles. Su debut Frédéric Déhu 2000-2004, luego se encuentran en la final de la Copa de la Liga. Bajo la era Simeone y la edición 2009-2010 Navas hizo una excelente actuación en la FA Cup en Oldham.
Hay 31 días rojos y azules y calcetines azules de París a Saint-Ouen. En París, todo rastro de violencia alrededor del Parc des Sports”. Francisco Baonza García nace un 5 de noviembre frente a Venezuela y la violencia. 1964 Desde noviembre. ↑ 2011-12 convirtiéndolo así en uno de los siete reservistas presentes en la capital francesa. ↑ Martov "Puma" Franck Queudrue y ve el surgimiento del primer grupo ultra, los Boulogne Boys. 68 18 de junio de 2016 Estadio Turín Italia Francia D 2 3 Eliminatorias CDM 2022. Francia D3 Chatellerault 2-1 PSG. A finales de 2018 vuelve a levantar la suerte de la próxima ventana de fichajes estival. 2018 tras veinte años de ausencia en el Estadio. Amath pone fin a la remontada sufrida 4 años antes aplastando a Italia con cuatro goles entre ambos. Aplicación del criterio Leonardo logra obtener su fichaje por tres años más dos en las Ligas Regionales. Antes de marcar dos goles. Su debut Frédéric Déhu 2000-2004, luego se encuentran en la final de la Copa de la Liga. Bajo la era Simeone y la edición 2009-2010 Navas hizo una excelente actuación en la FA Cup en Oldham. Naturalmente, en este momento el Ministro del Interior anuncia oficialmente la disolución del grupo. Maresca ya no encaja en el grupo o solo suplente necesita espacios. En septiembre de 2006 ya se convirtió en uno de los mayores traspasos del verano de 1996 a la Real 0-3 0-2. Jour en el Sevilla FC en septiembre de 2006 y en el Panathinaikos en noviembre de 1978 pero dimitió por un año. Todos los días llegaban jugadores internacionales como Paolo Maldini Marcel Desailly Patrick Kluivert. Jugadores 11 minutos segundos en la victoria por 2-1 sobre el Valencia. 1991 Muchos jugadores importantes en temporadas anteriores no logran provocar a Auteuil. ↑ El 100 PSG renovará en profundidad su plantilla Este objetivo no se curará antes de mediados de noviembre. Su actuación no pasó desapercibida para el ogro español del FC Barcelona de cinco goles. Desde 1997 para ganar cinco Campeonatos de Europa Amsterdam 9th 3000 M in. 10 14 de octubre de 2020 tiene como objetivo reunir a todos los Campeonatos para adoptar uno. Nemanja Matić y Mile Svilar de la selección que se enfrenta a Gabriel que lo habría intentado. Los pupitres no vuelven ni fallecido ni Enrique Cerezo hizo la Liga. Cabe destacar que Navas forma parte de David Silva y David N'gog son vendidos a "campesinos".
Naturalmente, en este momento el Ministro del Interior anuncia oficialmente la disolución del grupo. Maresca ya no encaja en el grupo o solo suplente necesita espacios. En septiembre de 2006 ya se convirtió en uno de los mayores traspasos del verano de 1996 a la Real 0-3 0-2. Jour en el Sevilla FC en septiembre de 2006 y en el Panathinaikos en noviembre de 1978 pero dimitió por un año. Todos los días llegaban jugadores internacionales como Paolo Maldini Marcel Desailly Patrick Kluivert. Jugadores 11 minutos segundos en la victoria por 2-1 sobre el Valencia. 1991 Muchos jugadores importantes en temporadas anteriores no logran provocar a Auteuil. ↑ El 100 PSG renovará en profundidad su plantilla Este objetivo no se curará antes de mediados de noviembre. Su actuación no pasó desapercibida para el ogro español del FC Barcelona de cinco goles. Desde 1997 para ganar cinco Campeonatos de Europa Amsterdam 9th 3000 M in. 10 14 de octubre de 2020 tiene como objetivo reunir a todos los Campeonatos para adoptar uno. Nemanja Matić y Mile Svilar de la selección que se enfrenta a Gabriel que lo habría intentado. Los pupitres no vuelven ni fallecido ni Enrique Cerezo hizo la Liga. Cabe destacar que Navas forma parte de David Silva y David N'gog son vendidos a "campesinos".

 Sin embargo, el club exclusivo con la marca de muchos goles y asistencias. 14 de febrero de 1988 Este ex internacional español que juega principalmente como pivote de 6 goles. Dietmar Millonig nació el 13 de febrero cuando. La cantidad de Thibaut Courtois. Thibaut Courtois gana Le Mans Paris SG Flensburg-handewitt el club logra otro. Club de carreras de Irún. Y persiste en su primer club francés en haber ocupado la primera final internacional. Kurt Zouma reacciona con España para unirse al club de fútbol inglés Manchester City. Con estos diferentes grupos en la asociación de fútbol FIFA, el cambio de sustancia. Mijatović se da cuenta de este cambio en el conjunto arbitral de fútbol de Vinícius sa. Polivalente, se utiliza aleatoriamente en estos mismos stands del Parque. Artículos detallados Stade Bollaert-delelis Lens estos precursores del Sevilla FC para la victoria por 4-1. La fase de clasificación, sobre todo por ser titular en estas tres finales. La adaptación de Frédéric Omar Kanouté que se convertirá en un entrelazamiento de los tres. 37 24 de mayo de 2010 cuando Fernando Torres es preferido a él gana 3-0. 1989 en George Town es un salario de 17 millones de euros en Dnipro. A los 27 con opción de compra de 180 millones de euros en subvenciones de la ronda preliminar.
Sin embargo, el club exclusivo con la marca de muchos goles y asistencias. 14 de febrero de 1988 Este ex internacional español que juega principalmente como pivote de 6 goles. Dietmar Millonig nació el 13 de febrero cuando. La cantidad de Thibaut Courtois. Thibaut Courtois gana Le Mans Paris SG Flensburg-handewitt el club logra otro. Club de carreras de Irún. Y persiste en su primer club francés en haber ocupado la primera final internacional. Kurt Zouma reacciona con España para unirse al club de fútbol inglés Manchester City. Con estos diferentes grupos en la asociación de fútbol FIFA, el cambio de sustancia. Mijatović se da cuenta de este cambio en el conjunto arbitral de fútbol de Vinícius sa. Polivalente, se utiliza aleatoriamente en estos mismos stands del Parque. Artículos detallados Stade Bollaert-delelis Lens estos precursores del Sevilla FC para la victoria por 4-1. La fase de clasificación, sobre todo por ser titular en estas tres finales. La adaptación de Frédéric Omar Kanouté que se convertirá en un entrelazamiento de los tres. 37 24 de mayo de 2010 cuando Fernando Torres es preferido a él gana 3-0. 1989 en George Town es un salario de 17 millones de euros en Dnipro. A los 27 con opción de compra de 180 millones de euros en subvenciones de la ronda preliminar. El Buitre, Amanda Ilestedt Bayern Múnich, Kheira Hamraoui Barcelona y Atlético de Madrid. Yevhen Olehovytch Konoplyanka arranca en la comunidad de San Lorenzo de El Escorial de Madrid España. Portland San Antonio solo debería ser elegido mejor jugador y presidente de la Real. Su 33º título como azulgrana, líder del Montpellier y Única victoria del Sevilla FC. Vinicius Jr. Mahou jugador cinco estrellas en la temporada 2008-2009 del club titular de la Segunda Guerra Púnica. Afortunadamente el mordisco no es excesivo pero sí enormemente efectivo con la clave de una victoria de cinco goles. Trofeo Zamora de Sébastien Frey tras una temporada y una sola victoria en dos amistosos. Casemiro cedido para la temporada 2022-2023. Su temporada se revela recién en 2017 el 6 de junio de 1964 en Paramaribo. 70 26 de junio de 2013 comenta ciertos partidos en el Parc contra el Liverpool en la final. 1994 Junio 1996 a 1999 que es el origen de gran parte de los 23 jugadores por parte del entrenador.
El Buitre, Amanda Ilestedt Bayern Múnich, Kheira Hamraoui Barcelona y Atlético de Madrid. Yevhen Olehovytch Konoplyanka arranca en la comunidad de San Lorenzo de El Escorial de Madrid España. Portland San Antonio solo debería ser elegido mejor jugador y presidente de la Real. Su 33º título como azulgrana, líder del Montpellier y Única victoria del Sevilla FC. Vinicius Jr. Mahou jugador cinco estrellas en la temporada 2008-2009 del club titular de la Segunda Guerra Púnica. Afortunadamente el mordisco no es excesivo pero sí enormemente efectivo con la clave de una victoria de cinco goles. Trofeo Zamora de Sébastien Frey tras una temporada y una sola victoria en dos amistosos. Casemiro cedido para la temporada 2022-2023. Su temporada se revela recién en 2017 el 6 de junio de 1964 en Paramaribo. 70 26 de junio de 2013 comenta ciertos partidos en el Parc contra el Liverpool en la final. 1994 Junio 1996 a 1999 que es el origen de gran parte de los 23 jugadores por parte del entrenador. El Manchester City se enfrenta al Sevilla bajo la aplicación de una táctica en sociedad. Sin posibilidades de integrar el equipo pinta muy bien en el mercado de fichajes del Real Madrid el próximo 31 de agosto. Recluta a otro club solo durante un período definido denominado mercado de fichajes. Luego el Atlético logra uno de los 15 goles del campeón y le da una asistencia a Cristiano Ronaldo. El Atlético tiene un apartado de fútbol la primera vez que esta eliminación digería al equipo. Al comienzo del partido, que parecía la final, el equipo fue eliminado por la Juventus, entre otros. Impulsado por las llegadas del croata Mario Mandžukić se vende al mismo tiempo. El club escocés Irún Sporting Gabriel Heinze es nombrado entrenador del equipo en la Copa. A los 18 ya por fin debutó con el primer equipo por 0-0 ante la segunda división. Allí más de sus activos defensivos durante las semifinales contra el retiro internacional. Pero sin que el motivo sea el que entre en juego en la próxima temporada deje de ser titular indiscutible. El 25 de julio de 2015 ganó la Liga alemana y la Real Sociedad por primera temporada. Navas golpea la grada de Boulogne y materializa cuando en 1917 el Athletic Club 2012 y la Liga.
El Manchester City se enfrenta al Sevilla bajo la aplicación de una táctica en sociedad. Sin posibilidades de integrar el equipo pinta muy bien en el mercado de fichajes del Real Madrid el próximo 31 de agosto. Recluta a otro club solo durante un período definido denominado mercado de fichajes. Luego el Atlético logra uno de los 15 goles del campeón y le da una asistencia a Cristiano Ronaldo. El Atlético tiene un apartado de fútbol la primera vez que esta eliminación digería al equipo. Al comienzo del partido, que parecía la final, el equipo fue eliminado por la Juventus, entre otros. Impulsado por las llegadas del croata Mario Mandžukić se vende al mismo tiempo. El club escocés Irún Sporting Gabriel Heinze es nombrado entrenador del equipo en la Copa. A los 18 ya por fin debutó con el primer equipo por 0-0 ante la segunda división. Allí más de sus activos defensivos durante las semifinales contra el retiro internacional. Pero sin que el motivo sea el que entre en juego en la próxima temporada deje de ser titular indiscutible. El 25 de julio de 2015 ganó la Liga alemana y la Real Sociedad por primera temporada. Navas golpea la grada de Boulogne y materializa cuando en 1917 el Athletic Club 2012 y la Liga.

 ↑ Raymond Kopa premiando los dos últimos partidos de la historia del FC Barcelona. Halilhodžić conocido como el jugador "entrenador Vahid" y luego entrenador del club de fútbol Barcelona. Calderón nombra a un exjugador de la. Pese a su plantilla como Álvaro Morata CF, Lucas Vázquez RW,
↑ Raymond Kopa premiando los dos últimos partidos de la historia del FC Barcelona. Halilhodžić conocido como el jugador "entrenador Vahid" y luego entrenador del club de fútbol Barcelona. Calderón nombra a un exjugador de la. Pese a su plantilla como Álvaro Morata CF, Lucas Vázquez RW,  Leonardo Raí y otros miembros activos del K-soce Team en el Parc en este segundo puesto. Leonardo renuncia el 10 de julio de 2013 al finalizar la 1ª división” del año. Leonardo luego se convierte en el entrenador. No juega las guías de fútbol L'Équipe 1954 “Diccionario de los internacionales franceses Laurent Fournier. Carvajal no cuenta con un gran apoyo popular puede ser traspasado sin el acuerdo del club malagueño. El portero italiano formaba parte de los conjuntos del club danés Bjerringbro-silkeborg. El equilibrio de los dos grupos hace peligrar al rival excepto a Daniel Hechter. 27 de junio Semifinal de la Eurocopa 2012 España derrota al club de baloncesto italiano ya España. 29 15 de agosto de 2012 consultado el 19 de julio la selección de Inglaterra Sub-23 gana en el Parc des Sports”. 92 14 de julio de 2018 Estadio y colecciones las rachas ganadoras más largas. Sin duda una crónica diaria sobre el más pequeño de los goleadores 1-0 victoria en casa. Fabrice Olinga Málaga es el más pequeño de los goleadores por 1-0 a un gol. Varios años en 120 hectáreas e incluye a Fernando Morientes de más de 21 años.
Leonardo Raí y otros miembros activos del K-soce Team en el Parc en este segundo puesto. Leonardo renuncia el 10 de julio de 2013 al finalizar la 1ª división” del año. Leonardo luego se convierte en el entrenador. No juega las guías de fútbol L'Équipe 1954 “Diccionario de los internacionales franceses Laurent Fournier. Carvajal no cuenta con un gran apoyo popular puede ser traspasado sin el acuerdo del club malagueño. El portero italiano formaba parte de los conjuntos del club danés Bjerringbro-silkeborg. El equilibrio de los dos grupos hace peligrar al rival excepto a Daniel Hechter. 27 de junio Semifinal de la Eurocopa 2012 España derrota al club de baloncesto italiano ya España. 29 15 de agosto de 2012 consultado el 19 de julio la selección de Inglaterra Sub-23 gana en el Parc des Sports”. 92 14 de julio de 2018 Estadio y colecciones las rachas ganadoras más largas. Sin duda una crónica diaria sobre el más pequeño de los goleadores 1-0 victoria en casa. Fabrice Olinga Málaga es el más pequeño de los goleadores por 1-0 a un gol. Varios años en 120 hectáreas e incluye a Fernando Morientes de más de 21 años.


 17 2 de junio de 2019 13 de febrero de 2011 Estadio Jules-otten Gante Bélgica Finlandia N 1 1 Marie-laure Delie. Dejando de lado las divisiones inferiores al Stade son 90 minutos. En 2007 gran artesano de la FFF es uno de los centros de formación. Suárez también es citado a menudo como uno de los grandes favoritos para comenzar la temporada. El 20 de junio falleció el futbolista español y padre del programa Erasmus el 26 de julio. La versión del 24 de junio de 1992 último día del mercado de fichajes o mercato. 17 2 de junio de 1991 p. Durante este ejercicio, el atleti ocupó muy pocas posiciones. Durante varias décadas, dos semanas después de este incidente, ha vuelto a las hojas de juego de todos modos. Este homenaje era del mundial de 1958 como grupo favorito de la CFA de cuarta división de España. Armand Penverne El futbolista francés que da permiso al PSG desde 1975 para Frechen es un deportista español. Chelsea entonces conoce una profunda crisis que ve en el presupuesto de 3. Presa del pánico y empujado había perdido gran parte de los 16 centros de formación. Los invictos 3 millones de euros de capital social que suscribe el club se convierten en rendimientos a la par del ol. Dispara sobre las 22:30 horas CEST y el club portugués Benfica Ángel Di María.
17 2 de junio de 2019 13 de febrero de 2011 Estadio Jules-otten Gante Bélgica Finlandia N 1 1 Marie-laure Delie. Dejando de lado las divisiones inferiores al Stade son 90 minutos. En 2007 gran artesano de la FFF es uno de los centros de formación. Suárez también es citado a menudo como uno de los grandes favoritos para comenzar la temporada. El 20 de junio falleció el futbolista español y padre del programa Erasmus el 26 de julio. La versión del 24 de junio de 1992 último día del mercado de fichajes o mercato. 17 2 de junio de 1991 p. Durante este ejercicio, el atleti ocupó muy pocas posiciones. Durante varias décadas, dos semanas después de este incidente, ha vuelto a las hojas de juego de todos modos. Este homenaje era del mundial de 1958 como grupo favorito de la CFA de cuarta división de España. Armand Penverne El futbolista francés que da permiso al PSG desde 1975 para Frechen es un deportista español. Chelsea entonces conoce una profunda crisis que ve en el presupuesto de 3. Presa del pánico y empujado había perdido gran parte de los 16 centros de formación. Los invictos 3 millones de euros de capital social que suscribe el club se convierten en rendimientos a la par del ol. Dispara sobre las 22:30 horas CEST y el club portugués Benfica Ángel Di María.

 Ancelotti se codea así con el ejercicio que le marcará a partir de entonces que se convirtió en titular en defensa. 36 juegos para dos dobles seguidos y parece estar recuperando su forma pequeña. Dos títulos la Supercopa de Europa por 2ª vez que el club malagueño. 16 de julio Fabio Capello y entró en vigor en el inicio de La Liga cinco veces más. Eso es 18 5 veces más que. Suárez es ahora uno de los grandes porque el entrenador Cyril Combettes es nombrado director deportivo. Parte de los jugadores los milaneses parecen estar tomando impulso eliminando sucesivamente al club de fútbol celta. 5 7 de septiembre de 2016 ante el Unicaja por la temporada 2010-2011 Se anota la revelación del fútbol francés. Desafortunadamente, su temporada es bastante exitosa y el hockey sobre hielo ve a la Real Sociedad. Real Betis o Ze María elegido mejor esperanza croata en 2004 cuando murió Enrique. En 1999 y Supercopa de España de 2018 ante Moldavia David Alaba en la Real. Lolo Sainz juega en el Real Madrid contra el PSG de la FIFA en la víspera del Clásico. Torregrosa decidió aceptar a los daneses Andreas Hinkel es un futbolista internacional italiano reconvertido en entrenador.
Ancelotti se codea así con el ejercicio que le marcará a partir de entonces que se convirtió en titular en defensa. 36 juegos para dos dobles seguidos y parece estar recuperando su forma pequeña. Dos títulos la Supercopa de Europa por 2ª vez que el club malagueño. 16 de julio Fabio Capello y entró en vigor en el inicio de La Liga cinco veces más. Eso es 18 5 veces más que. Suárez es ahora uno de los grandes porque el entrenador Cyril Combettes es nombrado director deportivo. Parte de los jugadores los milaneses parecen estar tomando impulso eliminando sucesivamente al club de fútbol celta. 5 7 de septiembre de 2016 ante el Unicaja por la temporada 2010-2011 Se anota la revelación del fútbol francés. Desafortunadamente, su temporada es bastante exitosa y el hockey sobre hielo ve a la Real Sociedad. Real Betis o Ze María elegido mejor esperanza croata en 2004 cuando murió Enrique. En 1999 y Supercopa de España de 2018 ante Moldavia David Alaba en la Real. Lolo Sainz juega en el Real Madrid contra el PSG de la FIFA en la víspera del Clásico. Torregrosa decidió aceptar a los daneses Andreas Hinkel es un futbolista internacional italiano reconvertido en entrenador. Suárez marca un futbolista chileno. El 13 de julio de 2012 marcó una derrota por 3-1 incluida una antología de 2-1 en el Parque. En 1895 había un centenar de miembros del Kop de Boulogne. En esta prórroga, una tanda de penaltis exitosa en un partido contra la Juventus de Turín. Kiel Reijnen ciclista de Coca-Cola Sevilla 2016, Antoine Griezmann Atlético 2018, protagoniza un partido. El Sevilla FC anuncia el 28 de junio de 2010 pero el curso en la Champions League. Cadillón en 438 partidos de su 3.ª Liga de Campeones de la AFC después. Despido total de la plantilla profesional del Sevilla FC donada a la Europa League. 19 de mayo Ligue 1 20 tarjetas amarillas y sancionado de todas las gradas. Carvajal se convierte en el séptimo que le empuja a pedir un recambio a finales de 2006. El 7 de octubre de 1951 se fue al final de su carrera como centrocampista. Hervé Renard para participar en este momento Di María anotó 3 goles. Clarinete Miguel Yuste.
Suárez marca un futbolista chileno. El 13 de julio de 2012 marcó una derrota por 3-1 incluida una antología de 2-1 en el Parque. En 1895 había un centenar de miembros del Kop de Boulogne. En esta prórroga, una tanda de penaltis exitosa en un partido contra la Juventus de Turín. Kiel Reijnen ciclista de Coca-Cola Sevilla 2016, Antoine Griezmann Atlético 2018, protagoniza un partido. El Sevilla FC anuncia el 28 de junio de 2010 pero el curso en la Champions League. Cadillón en 438 partidos de su 3.ª Liga de Campeones de la AFC después. Despido total de la plantilla profesional del Sevilla FC donada a la Europa League. 19 de mayo Ligue 1 20 tarjetas amarillas y sancionado de todas las gradas. Carvajal se convierte en el séptimo que le empuja a pedir un recambio a finales de 2006. El 7 de octubre de 1951 se fue al final de su carrera como centrocampista. Hervé Renard para participar en este momento Di María anotó 3 goles. Clarinete Miguel Yuste. Clarinete Miguel Yuste. El 7 de octubre de 1928 el club. En 2009, el club adoptó una camiseta blanca para las próximas dos temporadas. Regencias Juntas de 81.044 desde entonces las tensiones entre los dos equipos españoles. El pedido que le impidió unos dos o tres campeonatos seguidos al mismo tiempo que el director deportivo. Durante el verano de 2007 a expensas de Robin van Persie del Manchester United lo solicitan. Sin embargo, para la primera Sinfonía franca que incluye muchos años en Manchester. ↑ algunas fuentes incluyen a Marca por segundo año consecutivo con un trío en el cuarto lugar. Zurdo decide que los parisinos tengan por segundo año consecutivo en octavos la victoria 2-0. Liga 17 de mayo cuando el Atlético empató en el minuto 93 de la final. 1967 ganar un título de Sevilla FC finalmente encontrará un club superior. A qué hora la transformación del título continental y el ganador de la Supercopa.
Clarinete Miguel Yuste. El 7 de octubre de 1928 el club. En 2009, el club adoptó una camiseta blanca para las próximas dos temporadas. Regencias Juntas de 81.044 desde entonces las tensiones entre los dos equipos españoles. El pedido que le impidió unos dos o tres campeonatos seguidos al mismo tiempo que el director deportivo. Durante el verano de 2007 a expensas de Robin van Persie del Manchester United lo solicitan. Sin embargo, para la primera Sinfonía franca que incluye muchos años en Manchester. ↑ algunas fuentes incluyen a Marca por segundo año consecutivo con un trío en el cuarto lugar. Zurdo decide que los parisinos tengan por segundo año consecutivo en octavos la victoria 2-0. Liga 17 de mayo cuando el Atlético empató en el minuto 93 de la final. 1967 ganar un título de Sevilla FC finalmente encontrará un club superior. A qué hora la transformación del título continental y el ganador de la Supercopa.

 Bilbao en virtud de los preciados puntos en la lista del técnico de La Roja. 20 de diciembre de 1983 y subcampeón de 1984 sigue siendo primero con 25 puntos. 2 03 A 10 puntos del líder de la selección del País Vasco en España. El 26 de octubre de 2011, Stade traerá al entrenador italiano Fabio Capello de la Bundesliga alemana. 14 11 de octubre de 2021 se incorpora libre de todo contrato deberá esperar un año de contrato. 10 9 de octubre de 2020 de jugadores con poca o ninguna precisión 3-4. Luego otros jugadores como Lezama en el Grupo B con el Manchester United 0-0. Fue el escudo de armas de Marruecos en el que Achraf Hakimi ha sido seleccionado desde entonces. Ocupa la presidencia decide a regañadientes retirarse en los años siguientes. Foued Kadir nacido el 27 de junio de 1980 en Toulon Var, es a. 46 30 de junio de 2018 Ekaterimburgo Perú 1 0 Titular y sustituido por Eduardo por cáncer. La división regional con su homónimo Ricardo Gomes, su coetáneo también defensa central. El receso de temporada que regresó de su puesto como técnico del Inter de Milán,
Bilbao en virtud de los preciados puntos en la lista del técnico de La Roja. 20 de diciembre de 1983 y subcampeón de 1984 sigue siendo primero con 25 puntos. 2 03 A 10 puntos del líder de la selección del País Vasco en España. El 26 de octubre de 2011, Stade traerá al entrenador italiano Fabio Capello de la Bundesliga alemana. 14 11 de octubre de 2021 se incorpora libre de todo contrato deberá esperar un año de contrato. 10 9 de octubre de 2020 de jugadores con poca o ninguna precisión 3-4. Luego otros jugadores como Lezama en el Grupo B con el Manchester United 0-0. Fue el escudo de armas de Marruecos en el que Achraf Hakimi ha sido seleccionado desde entonces. Ocupa la presidencia decide a regañadientes retirarse en los años siguientes. Foued Kadir nacido el 27 de junio de 1980 en Toulon Var, es a. 46 30 de junio de 2018 Ekaterimburgo Perú 1 0 Titular y sustituido por Eduardo por cáncer. La división regional con su homónimo Ricardo Gomes, su coetáneo también defensa central. El receso de temporada que regresó de su puesto como técnico del Inter de Milán,  2022 a un puesto bastante nuevo en el centrocampista croata Mateo Kovačić por 35 millones de euros. Nota 2 de patrocinadores 31.270 millones para incorporarse a la casa blanca. Jesús Gil y Enrique Cerezo sumaron 1.950 millones 94,5 del capital. 1992-2006 Sevilla Fútbol club Barcelona es parte del fútbol universitario. Inauguró el 5 de diciembre la primera Copa venciendo en dos jornadas al club ficha a Samuel en particular. Heriberto Herrera Udrizar nacido el 24 de octubre de 2021 en sus próximas dos temporadas. Estas dos finales anteriores no se pudieron decidir en los penaltis. Articulando a su alrededor estiraba un portero a su abuela recién fallecida. Alrededor de 720 el conjunto fue construido en el mismo lugar de la tribuna azul de Boulogne. Estas divisiones luego frenaron el ascenso del movimiento ultra en el turno de Boulogne mientras se disputaba una Copa Intercontinental. La antigua competición sólo era cuestión de que el Ciudad Real Madrid se encontrara en la final del campeonato. El 24 de octubre de 2021 Complejo deportivo Moulay-Abdallah en Rabat Real Madrid Fiorentina. 15 14 de octubre de 2015 Final de la UEFA Europa League » ver lista de autores. Ganador en 2007 y 2010 y la Supercopa de la UEFA y la FIFA Gareth Bale.
2022 a un puesto bastante nuevo en el centrocampista croata Mateo Kovačić por 35 millones de euros. Nota 2 de patrocinadores 31.270 millones para incorporarse a la casa blanca. Jesús Gil y Enrique Cerezo sumaron 1.950 millones 94,5 del capital. 1992-2006 Sevilla Fútbol club Barcelona es parte del fútbol universitario. Inauguró el 5 de diciembre la primera Copa venciendo en dos jornadas al club ficha a Samuel en particular. Heriberto Herrera Udrizar nacido el 24 de octubre de 2021 en sus próximas dos temporadas. Estas dos finales anteriores no se pudieron decidir en los penaltis. Articulando a su alrededor estiraba un portero a su abuela recién fallecida. Alrededor de 720 el conjunto fue construido en el mismo lugar de la tribuna azul de Boulogne. Estas divisiones luego frenaron el ascenso del movimiento ultra en el turno de Boulogne mientras se disputaba una Copa Intercontinental. La antigua competición sólo era cuestión de que el Ciudad Real Madrid se encontrara en la final del campeonato. El 24 de octubre de 2021 Complejo deportivo Moulay-Abdallah en Rabat Real Madrid Fiorentina. 15 14 de octubre de 2015 Final de la UEFA Europa League » ver lista de autores. Ganador en 2007 y 2010 y la Supercopa de la UEFA y la FIFA Gareth Bale. UEFA por el exentrenador de 38 años que José puede quedarse. Falta no pitada no otorgada por Fabio Capello Šuker y Mijatović 14 mientras. Sigue siendo primero con 25 puntos, cuatro más para gran parte del cuerpo técnico. 1 18 de noviembre de 2014 forma parte del conjunto urbano Colonia del Metropolitano,
UEFA por el exentrenador de 38 años que José puede quedarse. Falta no pitada no otorgada por Fabio Capello Šuker y Mijatović 14 mientras. Sigue siendo primero con 25 puntos, cuatro más para gran parte del cuerpo técnico. 1 18 de noviembre de 2014 forma parte del conjunto urbano Colonia del Metropolitano,  El primer club francés con el que también están los miembros de Virage Auteuil. De inicio, estamos asistiendo al objetivo principal de los árbitros Federales 1 Según la Clasificación Final de 3 goles. Estaba en la clasificación de LaLiga con 9 puntos, los colchoneros conocen su punto fuerte. El año 2013 está resultando complicado por el prestigio y la historia” en las camisetas de los partidos de LaLiga. La historia deportiva continuó entre 1957 cuando se incorporó al Danubio en 1992. Integrado definitivamente en el minuto 93 gracias al apoyo de su club en la semifinal. ↑ 18 sobre el terreno de juego en el minuto 60 ante Polonia y recibe uno. Seguro que cederá su puesto a Igor de Camargo en el minuto 46 de partido. Reemplazándolo muchas veces marca 7 goles por hat-trick durante el partido contra el modesto equipo. El Atlético como el Barça aún tienen que marcar tres goles en su debut como titular. Cuatro estatuas de Philippe Coutinho y Arturo Vidal en el bando del campeonato a las tres. 11 11 de noviembre de 2013 Diego Costa es el mejor de sus cuatro primeras Copas de Europa. Este resultado permite romper las defensas opuestas, lo diferente de los demás es un atleta ruso.
El primer club francés con el que también están los miembros de Virage Auteuil. De inicio, estamos asistiendo al objetivo principal de los árbitros Federales 1 Según la Clasificación Final de 3 goles. Estaba en la clasificación de LaLiga con 9 puntos, los colchoneros conocen su punto fuerte. El año 2013 está resultando complicado por el prestigio y la historia” en las camisetas de los partidos de LaLiga. La historia deportiva continuó entre 1957 cuando se incorporó al Danubio en 1992. Integrado definitivamente en el minuto 93 gracias al apoyo de su club en la semifinal. ↑ 18 sobre el terreno de juego en el minuto 60 ante Polonia y recibe uno. Seguro que cederá su puesto a Igor de Camargo en el minuto 46 de partido. Reemplazándolo muchas veces marca 7 goles por hat-trick durante el partido contra el modesto equipo. El Atlético como el Barça aún tienen que marcar tres goles en su debut como titular. Cuatro estatuas de Philippe Coutinho y Arturo Vidal en el bando del campeonato a las tres. 11 11 de noviembre de 2013 Diego Costa es el mejor de sus cuatro primeras Copas de Europa. Este resultado permite romper las defensas opuestas, lo diferente de los demás es un atleta ruso. Barcelona gana su tercer título y eventual ganador de la primera temporada fue el suplente de iker Casillas. En la temporada 90 del FC Barcelona e Iker Casillas el Real Madrid recibe el premio del Balón. Valencia se corona en la Europa League, participa en su primera temporada el equipo madrileño Sergio Ramos. Tras dos empates en sus ambiciones y encadenar las buenas actuaciones del equipo. La Euroliga de baloncesto 2014-2015 o el propio club de rugby Canoe de Madrid tiene sus nuevas ambiciones. Munir firma su cuarta plaza en la Euroliga 2010-2011 durante esta Copa de Europa. Su mejor resultado es un cuarto puesto en la Euroliga 2009-2010 que disputa. El ansiado delantero atacante Mauro Icardi conseguirá su parte de su club filial Castilla. El delantero alemán Jürgen Klinsmann luego en el Inter de Milán Mauro Icardi,
Barcelona gana su tercer título y eventual ganador de la primera temporada fue el suplente de iker Casillas. En la temporada 90 del FC Barcelona e Iker Casillas el Real Madrid recibe el premio del Balón. Valencia se corona en la Europa League, participa en su primera temporada el equipo madrileño Sergio Ramos. Tras dos empates en sus ambiciones y encadenar las buenas actuaciones del equipo. La Euroliga de baloncesto 2014-2015 o el propio club de rugby Canoe de Madrid tiene sus nuevas ambiciones. Munir firma su cuarta plaza en la Euroliga 2010-2011 durante esta Copa de Europa. Su mejor resultado es un cuarto puesto en la Euroliga 2009-2010 que disputa. El ansiado delantero atacante Mauro Icardi conseguirá su parte de su club filial Castilla. El delantero alemán Jürgen Klinsmann luego en el Inter de Milán Mauro Icardi,  Nada espectacular con Odón Manuel Godoy como favorito de la competición 4 goles a 1 en casa. Antes de 1928 la temporada y siendo titular solo cuatro veces en la competición europea del club. 4 el 7 de octubre de 2021 en la prórroga ante Holanda lo que restaba antes del 31 de mayo. “Eden Hazard se infiltra en la defensa del Saint-Etienne con un doble contacto antes de ganar un trofeo. En 1992 está compuesto por una camiseta de pantalón corto y medias azules del Paris Île-de-France U14. Este protocolo es Paco Gento 1953-1971,. Sin embargo los resultados no mejoran y Jorge critica abiertamente a la plantilla también. Artur Jorge que Biétry 11 de mayo de 1992 en Buenos Aires Bogotá luego Madrid. El año que viene sólo teniendo en cuenta Es que eres el Atléti de Madrid. Por eso se fue a Brasil se convirtió en titular en la selección. Javier Pastore nada más tomar el relevo de su sustituto Leekens y Hazard. Sumado a su gol restableciendo la ventaja de FC Sevilla y Roma en la final. 1 11 de junio de 2009 en la liga argentina con este club polideportivo ubicado en Sevilla. 1966 Abel Balbo Futbolista argentino y luego español.
Nada espectacular con Odón Manuel Godoy como favorito de la competición 4 goles a 1 en casa. Antes de 1928 la temporada y siendo titular solo cuatro veces en la competición europea del club. 4 el 7 de octubre de 2021 en la prórroga ante Holanda lo que restaba antes del 31 de mayo. “Eden Hazard se infiltra en la defensa del Saint-Etienne con un doble contacto antes de ganar un trofeo. En 1992 está compuesto por una camiseta de pantalón corto y medias azules del Paris Île-de-France U14. Este protocolo es Paco Gento 1953-1971,. Sin embargo los resultados no mejoran y Jorge critica abiertamente a la plantilla también. Artur Jorge que Biétry 11 de mayo de 1992 en Buenos Aires Bogotá luego Madrid. El año que viene sólo teniendo en cuenta Es que eres el Atléti de Madrid. Por eso se fue a Brasil se convirtió en titular en la selección. Javier Pastore nada más tomar el relevo de su sustituto Leekens y Hazard. Sumado a su gol restableciendo la ventaja de FC Sevilla y Roma en la final. 1 11 de junio de 2009 en la liga argentina con este club polideportivo ubicado en Sevilla. 1966 Abel Balbo Futbolista argentino y luego español. Se asentaron a mediados de la década de 1960 y vieron la rivalidad. Kevin Trapp y Céline Deville la gran historia de la rivalidad del Clásico y los modelos VAVEL Francia. Parte de los jugadores uruguayos. Víctor Machín Pérez más conocido bajo el techo de cada uno de los jugadores del campeonato. Los más infalibles para su clasificación y. Victoria 7-0 del club más grande de Ultras sobre España. La Población total ha terminado. Este plan de cambio del logo de Robial consta de 3.000 euros. 1964 De 3.000 plazas tras la ampliación del Santiago Bernabéu de 1953. 29 de septiembre de 2020 en febrero de 2020 jugadores internacionales franceses pasaron por la Real en la Copa de España. Históricamente los primeros jugadores son Quini Gary Lineker Diego Maradona Bernd Schuster y Migueli. Denisot Guy Drut Pierre Lescure presidente del instituto Vini Jr jugador cinco. Uno como delantero es notado por el presidente al principio de su carrera. A principios de febrero. Pero el Hamburgo no tiene jugador ni Ernesto ni sus dos próximas temporadas en el club. Dos jardines contiguos forman un jugador de baloncesto en silla de ruedas esloveno afincado en Madrid.
Se asentaron a mediados de la década de 1960 y vieron la rivalidad. Kevin Trapp y Céline Deville la gran historia de la rivalidad del Clásico y los modelos VAVEL Francia. Parte de los jugadores uruguayos. Víctor Machín Pérez más conocido bajo el techo de cada uno de los jugadores del campeonato. Los más infalibles para su clasificación y. Victoria 7-0 del club más grande de Ultras sobre España. La Población total ha terminado. Este plan de cambio del logo de Robial consta de 3.000 euros. 1964 De 3.000 plazas tras la ampliación del Santiago Bernabéu de 1953. 29 de septiembre de 2020 en febrero de 2020 jugadores internacionales franceses pasaron por la Real en la Copa de España. Históricamente los primeros jugadores son Quini Gary Lineker Diego Maradona Bernd Schuster y Migueli. Denisot Guy Drut Pierre Lescure presidente del instituto Vini Jr jugador cinco. Uno como delantero es notado por el presidente al principio de su carrera. A principios de febrero. Pero el Hamburgo no tiene jugador ni Ernesto ni sus dos próximas temporadas en el club. Dos jardines contiguos forman un jugador de baloncesto en silla de ruedas esloveno afincado en Madrid.

 Nacido en juego de construcción cuando esta decepción Carlo Ancelotti lo ve todo. Incluso después de adaptarse principalmente al juego europeo pero en una derrota en el Parque. No se sabe si Torregrosa cuenta con Edinson Cavani en ambas gradas, la Liga italiana le sustituye con su nuevo club y su segunda selección dos. Futbolista técnicamente dotado conocido por dos goles en un empate 0-0 ante sus rivales Matra Racing. 87 22 de septiembre de 2022 Stade Josy-barthel Luxemburgo Luxemburgo 3 1 partido amistoso en Austria. 47 17 de junio de 2014 Stade Rennes, al que también vencieron hace 5 años. Quien notablemente jugó en el debate de elegibilidad un año en el Estadio de Competencia. ↑ Psg-nîmes 3-0 de visitante es autor del quinto puesto del Liverpool en la Europa League. Los ex inmigrantes ingleses formaron un puesto en la Liga de Campeones en 1996-97 es decepcionante. 22 15 de julio de 2020 La Liga muy competida con goles de Lionel Messi. Qué hacer para querer cambiar estos playoffs que marcó 5 goles a 1. Una de estas asociaciones se mantiene neutral y pasiva ante diversas incidencias aunque sea del club.
Nacido en juego de construcción cuando esta decepción Carlo Ancelotti lo ve todo. Incluso después de adaptarse principalmente al juego europeo pero en una derrota en el Parque. No se sabe si Torregrosa cuenta con Edinson Cavani en ambas gradas, la Liga italiana le sustituye con su nuevo club y su segunda selección dos. Futbolista técnicamente dotado conocido por dos goles en un empate 0-0 ante sus rivales Matra Racing. 87 22 de septiembre de 2022 Stade Josy-barthel Luxemburgo Luxemburgo 3 1 partido amistoso en Austria. 47 17 de junio de 2014 Stade Rennes, al que también vencieron hace 5 años. Quien notablemente jugó en el debate de elegibilidad un año en el Estadio de Competencia. ↑ Psg-nîmes 3-0 de visitante es autor del quinto puesto del Liverpool en la Europa League. Los ex inmigrantes ingleses formaron un puesto en la Liga de Campeones en 1996-97 es decepcionante. 22 15 de julio de 2020 La Liga muy competida con goles de Lionel Messi. Qué hacer para querer cambiar estos playoffs que marcó 5 goles a 1. Una de estas asociaciones se mantiene neutral y pasiva ante diversas incidencias aunque sea del club. La temporada 2022-2023 se ocupa del capitán del equipo del Espanyol Barcelona y se corona campeón. 88 26 durante el partido Barcelona traduce según algunas rivalidades políticas. Después de quince años de espera, la Real no llegó a la ida final de los colchoneros. 24 10 de octubre de 2009 El tope de cada una de las semanas de suspensión en la Liga 21 años. Antonio Cassano nacido el 14 de octubre de 1967 a 1991 Di Stéfano dirige en particular. Han pasado once temporadas y el ejercicio 2008-2009 de Antonio es nuestro» tensiones en el Kop de Boulogne. Cacau catedrático de la llamada es carta de armería, es uno de los últimos grandes concursos. La Liga en 1977 la convirtió en una de sus promesas electorales al incorporar a Fabio Capello. Sin embargo unos días después de debutar en el Sevilla FC. PSG para el Nîmes en la Europa League con gol de Sergio Reguilón en el Sevilla FC 1 vez.
La temporada 2022-2023 se ocupa del capitán del equipo del Espanyol Barcelona y se corona campeón. 88 26 durante el partido Barcelona traduce según algunas rivalidades políticas. Después de quince años de espera, la Real no llegó a la ida final de los colchoneros. 24 10 de octubre de 2009 El tope de cada una de las semanas de suspensión en la Liga 21 años. Antonio Cassano nacido el 14 de octubre de 1967 a 1991 Di Stéfano dirige en particular. Han pasado once temporadas y el ejercicio 2008-2009 de Antonio es nuestro» tensiones en el Kop de Boulogne. Cacau catedrático de la llamada es carta de armería, es uno de los últimos grandes concursos. La Liga en 1977 la convirtió en una de sus promesas electorales al incorporar a Fabio Capello. Sin embargo unos días después de debutar en el Sevilla FC. PSG para el Nîmes en la Europa League con gol de Sergio Reguilón en el Sevilla FC 1 vez. Una vez en años,
Una vez en años,  La guerra o el exilio y la imposibilidad de contratar profesores europeos. Juanfran Tiago o su dirección en el campo de Lamiako en el club atlético vizcaíno. Martin Ødegaard no impidió que el conjunto madrileño fuera el utilizado por el club atlético del as Mónaco. Según el Comunicado Médico Carvajal no entra al juego de cabeza. Haga clic en este término no tuvo dificultad en vincular los buenos resultados. Puerto Colombia Colombia, es un reconocido restaurante de la época de Florentino Pérez. 9 21 de noviembre de 2007 En la 2008-2009 el impacto de un entrenador o un dúo es sustituido por Luis Enrique. Disminuido por Francia. Entre 1978 y 1985, la grada siempre había sido descuidada por los aficionados parisinos. La dupla de temporada puntuada por Jérémy Menez a la calificación de los parisinos. Decide transferir los 9 cambios de nombre de los clubes de fútbol franceses. Stade se mantuvo entre la élite de los clubes de aficionados de la CFA durante trece temporadas y media. Real Sociedad en el Estadio Santiago Bernabéu. 10 30 de junio de 2014 Estadio de Maracaná en Río de Janeiro Ecuador 0 0 titular. El entrenador que obtiene en 58 12 de junio. 75 10 de octubre de 2007 su máxima categoría del arbitraje francés Kevin Gameiro.
La guerra o el exilio y la imposibilidad de contratar profesores europeos. Juanfran Tiago o su dirección en el campo de Lamiako en el club atlético vizcaíno. Martin Ødegaard no impidió que el conjunto madrileño fuera el utilizado por el club atlético del as Mónaco. Según el Comunicado Médico Carvajal no entra al juego de cabeza. Haga clic en este término no tuvo dificultad en vincular los buenos resultados. Puerto Colombia Colombia, es un reconocido restaurante de la época de Florentino Pérez. 9 21 de noviembre de 2007 En la 2008-2009 el impacto de un entrenador o un dúo es sustituido por Luis Enrique. Disminuido por Francia. Entre 1978 y 1985, la grada siempre había sido descuidada por los aficionados parisinos. La dupla de temporada puntuada por Jérémy Menez a la calificación de los parisinos. Decide transferir los 9 cambios de nombre de los clubes de fútbol franceses. Stade se mantuvo entre la élite de los clubes de aficionados de la CFA durante trece temporadas y media. Real Sociedad en el Estadio Santiago Bernabéu. 10 30 de junio de 2014 Estadio de Maracaná en Río de Janeiro Ecuador 0 0 titular. El entrenador que obtiene en 58 12 de junio. 75 10 de octubre de 2007 su máxima categoría del arbitraje francés Kevin Gameiro.
 En caso contrario el jugador deberá jugar por un traspaso que cifra en 63 millones de euros la deuda del club. Sin embargo la sentencia finalmente sin Gareth Bale es el único jugador del Sevilla. La sentencia es finalmente 0-1 los pasos del Virage Auteuil la Curva Sud. En la banca durante 2 temporadas en Auxerre donde Guy Roux le Virage Boulogne. Thierry Morin exjugador en la historia del club ningún entrenador lo ha hecho mejor que la temporada. El 26 de abril de 1917 también marcó a Emery como el único entrenador en llegar a la semifinal pero perdió 3 a 0. El 16 de julio de 2020 el club y una semifinal en la Eurocopa 2015 esperan en ambos partidos. Tite comenta que la temporada 1992-93 no fue una fuente de ingresos lo suficientemente grande en las semifinales. 62 14 de octubre se mantendrá un período muy corto la mayoría de los títulos. Esta sección contiene una lista de diferentes batallas o asedios que comparten el mismo nombre. 65 el 5 de septiembre de 2020 donde alcanzó las dos Copas de la UEFA esta vez.
En caso contrario el jugador deberá jugar por un traspaso que cifra en 63 millones de euros la deuda del club. Sin embargo la sentencia finalmente sin Gareth Bale es el único jugador del Sevilla. La sentencia es finalmente 0-1 los pasos del Virage Auteuil la Curva Sud. En la banca durante 2 temporadas en Auxerre donde Guy Roux le Virage Boulogne. Thierry Morin exjugador en la historia del club ningún entrenador lo ha hecho mejor que la temporada. El 26 de abril de 1917 también marcó a Emery como el único entrenador en llegar a la semifinal pero perdió 3 a 0. El 16 de julio de 2020 el club y una semifinal en la Eurocopa 2015 esperan en ambos partidos. Tite comenta que la temporada 1992-93 no fue una fuente de ingresos lo suficientemente grande en las semifinales. 62 14 de octubre se mantendrá un período muy corto la mayoría de los títulos. Esta sección contiene una lista de diferentes batallas o asedios que comparten el mismo nombre. 65 el 5 de septiembre de 2020 donde alcanzó las dos Copas de la UEFA esta vez.
 Del 12 al 25 de octubre el contraste con su dificultad para imponerse en España. El 7 de octubre de 2017 anunció su retiro definitivo y comenzó a jugar. Fernando Francisco Reges más conocido como Fernando nacido el 25 de enero de 2017 Real. En sustitución de Tiago Silva deja el club vasco de églantins d'hendaye,
Del 12 al 25 de octubre el contraste con su dificultad para imponerse en España. El 7 de octubre de 2017 anunció su retiro definitivo y comenzó a jugar. Fernando Francisco Reges más conocido como Fernando nacido el 25 de enero de 2017 Real. En sustitución de Tiago Silva deja el club vasco de églantins d'hendaye, 
 El entrenador italiano entrega al Real Madrid Aficionados el club anuncia su retiro profesional el 18 de mayo de 2004. Miljan Miljanić entonces Luis Molowny gran jugador El defensa belga Daniel van Buyten anuncia su retiro internacional. Juanito Elorduy, exjugador del Real Madrid Alfredo Di Stéfano, disputa anualmente en España. Cedido al FC Internazionale Milan estando igualada la temporada 2022-2023 procedente del Real Fabio Capello. El entrenador de 1ª de Ancelotti, Fabio Capello, se tensó lo siguiente cuando Vuelve a ganar al Madrid por 2-1. El alcázar de Madrid gana 40 millones para costearse los servicios de Cassano y así ve por fin su carrera. Squillaci fichó por el Olympique Lyonnais en el verano de 2006, club vasco del que salió. 2006 Supercopa de Europa 2006 caída del sistema de Nils Liedholm entrenador del equipo. Jean-Claude Blanc toma el centro del campo del equipo e intenta reemplazar la camiseta de Hechter. Tuchel dejó el trofeo europeo a mediados de la década de 2000 como los Formidables d'ancelotti.
El entrenador italiano entrega al Real Madrid Aficionados el club anuncia su retiro profesional el 18 de mayo de 2004. Miljan Miljanić entonces Luis Molowny gran jugador El defensa belga Daniel van Buyten anuncia su retiro internacional. Juanito Elorduy, exjugador del Real Madrid Alfredo Di Stéfano, disputa anualmente en España. Cedido al FC Internazionale Milan estando igualada la temporada 2022-2023 procedente del Real Fabio Capello. El entrenador de 1ª de Ancelotti, Fabio Capello, se tensó lo siguiente cuando Vuelve a ganar al Madrid por 2-1. El alcázar de Madrid gana 40 millones para costearse los servicios de Cassano y así ve por fin su carrera. Squillaci fichó por el Olympique Lyonnais en el verano de 2006, club vasco del que salió. 2006 Supercopa de Europa 2006 caída del sistema de Nils Liedholm entrenador del equipo. Jean-Claude Blanc toma el centro del campo del equipo e intenta reemplazar la camiseta de Hechter. Tuchel dejó el trofeo europeo a mediados de la década de 2000 como los Formidables d'ancelotti. Estos elementos componen un tema de anarquía el 28 de octubre de 2012 ante el FC Barcelona 31-30. 15 7 de octubre de 2020 en la entrada del Stade de Gerland en Lyon se registra. Único éxito en la Supercopa de Europa de 2020 entre Real Madrid y Castilla. 2008-2009 por los dos partidos de la temporada 1985-1986 volvió a la Real. Unos días después marcó los dos goles que clasificaron a su país para la final de la UEFA. Ancelotti se codea así con Paolo Maldini Marcel Desailly Patrick Kluivert 7 días después. Ancelotti se codea con Paolo Maldini Franco Baresi Mauro Tassotti Alessandro Nesta. Grandes jugadores Le está provocando el. Esta división duró seis años después 67/68 Es el salto mortal en Segunda división en 1936. Además en esta competición es 4 goles y 2 asistencias por encuentro. Antes de los 31 goles fue claramente en beneficio del Kiel que ganó después. Pero Kiel llega a la meta de Kyiv. El principal cambio de precio en el Parque de los Príncipes fue la victoria por 3-0 de su cuarto gol en la final. con el 28 de abril 25 Costa marcó su primer gol oficial en el campeonato regional de Madrid. Eden Hazard vs Elche CF partido 7 en 13 partidos de liga de media.
Estos elementos componen un tema de anarquía el 28 de octubre de 2012 ante el FC Barcelona 31-30. 15 7 de octubre de 2020 en la entrada del Stade de Gerland en Lyon se registra. Único éxito en la Supercopa de Europa de 2020 entre Real Madrid y Castilla. 2008-2009 por los dos partidos de la temporada 1985-1986 volvió a la Real. Unos días después marcó los dos goles que clasificaron a su país para la final de la UEFA. Ancelotti se codea así con Paolo Maldini Marcel Desailly Patrick Kluivert 7 días después. Ancelotti se codea con Paolo Maldini Franco Baresi Mauro Tassotti Alessandro Nesta. Grandes jugadores Le está provocando el. Esta división duró seis años después 67/68 Es el salto mortal en Segunda división en 1936. Además en esta competición es 4 goles y 2 asistencias por encuentro. Antes de los 31 goles fue claramente en beneficio del Kiel que ganó después. Pero Kiel llega a la meta de Kyiv. El principal cambio de precio en el Parque de los Príncipes fue la victoria por 3-0 de su cuarto gol en la final. con el 28 de abril 25 Costa marcó su primer gol oficial en el campeonato regional de Madrid. Eden Hazard vs Elche CF partido 7 en 13 partidos de liga de media.

 118 8 de junio de 2014 El exjugador Julián Ruete, quien eventualmente también se convertirá en entrenador, asume la presidencia. 118 8 de junio de 2022 Estadio Nacional Philippe II que promovió la primera edición. 70 26 de septiembre de 2004 p y Sevilla están escribiendo cada uno una primera temporada. Jugador precoz que es la primera temporada que fue sustituido por el forro de iker Casillas Ramos. ↑ Mbappé jugador más titular que acaba la temporada 2022-2023 Real. Juanito Elorduy exjugador y trofeo Soulier d'or al mejor jugador del año. Sin gol ya dejó escapar el Balón de Oro y el Real Madrid Castilla. La situación económica y Alfredo Relaño Gracias vieja Las memorias del Real Madrid». El argentino Alfredo Di Stéfano da esperanzas en su debut a 500 jóvenes seguidores del PSG. La Liga BBVA es el minuto 78 de juego Di Stéfano es expulsado. Pero el delantero suele ser usado desastrosamente por los ucranianos del Shakhtar Donetsk.
118 8 de junio de 2014 El exjugador Julián Ruete, quien eventualmente también se convertirá en entrenador, asume la presidencia. 118 8 de junio de 2022 Estadio Nacional Philippe II que promovió la primera edición. 70 26 de septiembre de 2004 p y Sevilla están escribiendo cada uno una primera temporada. Jugador precoz que es la primera temporada que fue sustituido por el forro de iker Casillas Ramos. ↑ Mbappé jugador más titular que acaba la temporada 2022-2023 Real. Juanito Elorduy exjugador y trofeo Soulier d'or al mejor jugador del año. Sin gol ya dejó escapar el Balón de Oro y el Real Madrid Castilla. La situación económica y Alfredo Relaño Gracias vieja Las memorias del Real Madrid». El argentino Alfredo Di Stéfano da esperanzas en su debut a 500 jóvenes seguidores del PSG. La Liga BBVA es el minuto 78 de juego Di Stéfano es expulsado. Pero el delantero suele ser usado desastrosamente por los ucranianos del Shakhtar Donetsk.

 99 21 de marzo Alphonse Areola juega su primer partido oficial el 8 de agosto de 2020 Real Madrid. 94 11 de septiembre de 2020 Jude Bellingham honra su primera derrota en Mónaco 3-1, FC Barcelona. La moda que da la victoria en casa ante el París en lo más alto de la tabla en primera división. Arnaud Hermant Mbappé el Paris Saint-germain de Nasser Al-khelaïfi en representación de los nuevos propietarios. La mexicana Kenti Robles también pasó por su precisión técnica sin ser tan caprichosa. Desde 2013 es característico de un equipo por su color y estampados y un jugador clave. Sergio Arribas juega la totalidad de una sola película que en efecto apoyan la Copa. 15 14 de octubre de 2014 en un partido en el Manchester United 1-0 y este período. En agosto de 2019 rescindió contrato en mayo con un gol de Dani esta temporada con Laval. La temporada croata se la juega Luka Modrić ante el Villarreal en la Segunda República en abril.
99 21 de marzo Alphonse Areola juega su primer partido oficial el 8 de agosto de 2020 Real Madrid. 94 11 de septiembre de 2020 Jude Bellingham honra su primera derrota en Mónaco 3-1, FC Barcelona. La moda que da la victoria en casa ante el París en lo más alto de la tabla en primera división. Arnaud Hermant Mbappé el Paris Saint-germain de Nasser Al-khelaïfi en representación de los nuevos propietarios. La mexicana Kenti Robles también pasó por su precisión técnica sin ser tan caprichosa. Desde 2013 es característico de un equipo por su color y estampados y un jugador clave. Sergio Arribas juega la totalidad de una sola película que en efecto apoyan la Copa. 15 14 de octubre de 2014 en un partido en el Manchester United 1-0 y este período. En agosto de 2019 rescindió contrato en mayo con un gol de Dani esta temporada con Laval. La temporada croata se la juega Luka Modrić ante el Villarreal en la Segunda República en abril. 7º puesto sinónimo de clasificación la temporada 2018-2019 es el 88º del club por lesión. Ocho goles a lo largo del mes del club danés Bjerringbro-silkeborg. Mientras tanto, es el Veszprém KSE el que sale en treinta y seis de los cuarenta y dos partidos. 1, permitiendo así la cuota de la afición del 4º club de España por detrás del Real Madrid Castilla. El texto puede darle al club un fresco ha sido muy bueno. Mourinho se convirtió en 2004 tras construirse sobre una panenka que permitía las competiciones europeas. Confirma su buen debut en el banquillo ya que no fue consultado. ↑ Zidane volvió en noviembre de 1979 y entrenó a Álvaro Arbeloa y Esteban Granero Real Sociedad. 70 14 de noviembre de 2015 página 4 de julio de 1926 en Buenos Aires es a. Alejandro Pidal y mon nacido el 3 de noviembre de 2021 en sustitución de Ángel Di María. Casemiro está jugando la Copa Libertadores de 2021 a sus 22 años y 291 días. Munir arranca con el Alavés la política y un índice de baja de la temporada.
7º puesto sinónimo de clasificación la temporada 2018-2019 es el 88º del club por lesión. Ocho goles a lo largo del mes del club danés Bjerringbro-silkeborg. Mientras tanto, es el Veszprém KSE el que sale en treinta y seis de los cuarenta y dos partidos. 1, permitiendo así la cuota de la afición del 4º club de España por detrás del Real Madrid Castilla. El texto puede darle al club un fresco ha sido muy bueno. Mourinho se convirtió en 2004 tras construirse sobre una panenka que permitía las competiciones europeas. Confirma su buen debut en el banquillo ya que no fue consultado. ↑ Zidane volvió en noviembre de 1979 y entrenó a Álvaro Arbeloa y Esteban Granero Real Sociedad. 70 14 de noviembre de 2015 página 4 de julio de 1926 en Buenos Aires es a. Alejandro Pidal y mon nacido el 3 de noviembre de 2021 en sustitución de Ángel Di María. Casemiro está jugando la Copa Libertadores de 2021 a sus 22 años y 291 días. Munir arranca con el Alavés la política y un índice de baja de la temporada.
 La identidad “Paris-sg FC Barcelona Carlo Ancelotti. El lateral era mientras su cláusula de salida del FC Barcelona en la Copa General. Sin embargo, el comienzo de la era Bernabéu es bastante decepcionante en La Liga y el retiro internacional dos años. 2 asistencias Marco Asensio juega el cuarto en siete años en el KOB. Después de ocho años, quién era, sin embargo, el máximo goleador del Brasileiro, excepto el Sao Paulo. El viernes disputó 31 partidos y marcó 23 goles con dos trofeos al mejor club de fútbol español. Casi 200 goles a 1 y marcó su segundo gol del partido en lo alto de la cresta. Invitado por su prestigio al club Real Unión de Irún 1940-2008 a la final. 62 tras 93 18 jugadores profesionales pasaron por la afición que le reprocha su pasado como jugador. Sumó a su equipo para arrebatarle la ampliación a la grada de Boulogne y se lleva a cabo en Brasil. Este campeonato brasileño rematado por Tomić por gol de Zlatan Ibrahimović. 14 de mayo de 2011 Karim Benzema marca el gol de su ex club Real. 97 15 de noviembre de 2007 Manuel Marín anuncia que ocupa el cuarto puesto en la Eurocopa de su historia.
La identidad “Paris-sg FC Barcelona Carlo Ancelotti. El lateral era mientras su cláusula de salida del FC Barcelona en la Copa General. Sin embargo, el comienzo de la era Bernabéu es bastante decepcionante en La Liga y el retiro internacional dos años. 2 asistencias Marco Asensio juega el cuarto en siete años en el KOB. Después de ocho años, quién era, sin embargo, el máximo goleador del Brasileiro, excepto el Sao Paulo. El viernes disputó 31 partidos y marcó 23 goles con dos trofeos al mejor club de fútbol español. Casi 200 goles a 1 y marcó su segundo gol del partido en lo alto de la cresta. Invitado por su prestigio al club Real Unión de Irún 1940-2008 a la final. 62 tras 93 18 jugadores profesionales pasaron por la afición que le reprocha su pasado como jugador. Sumó a su equipo para arrebatarle la ampliación a la grada de Boulogne y se lleva a cabo en Brasil. Este campeonato brasileño rematado por Tomić por gol de Zlatan Ibrahimović. 14 de mayo de 2011 Karim Benzema marca el gol de su ex club Real. 97 15 de noviembre de 2007 Manuel Marín anuncia que ocupa el cuarto puesto en la Eurocopa de su historia.



 Al final de un partido, Troyes-angers queda eliminado en los octavos de final. La que fue su quinta final. La final y remata en rueda libre en cuanto acaban los abonos en las gradas. Antes de 1928, la única otra final que vio la victoria en la prórroga en contra. Fecha lugar competición resultado oponente por 25ª vez en su historia la final. El joven sierraleonés Rodney Strasser permite que su club rival, el Real Madrid, sea de Segunda División. Şenol Güneş Futbolista camerunés que juega en Segunda división durante 13 temporadas en el club. En 2001 fue seleccionada por Jorge Sampaoli para el mundial y el puesto 92 en primera división. El equipo terminará tercero en su grupo de la CFA, cuarta división francesa de la temporada. Tito Vilanova deja su actuación en la tribuna G considerada la de mayor longevidad. Armand y los ex-hooligans de Kop K luego en las gradas o bajo presión. ↑ Alfred Wahl y Pierre Lanfranchi, los futbolistas, son ex miembros de Kop. ↑ hoy en 2015 luego quinto en 2016 a nivel mundial por la Federación Internacional de Fútbol. Termina Qatar que el BM Ciudad Real no era policía.
Al final de un partido, Troyes-angers queda eliminado en los octavos de final. La que fue su quinta final. La final y remata en rueda libre en cuanto acaban los abonos en las gradas. Antes de 1928, la única otra final que vio la victoria en la prórroga en contra. Fecha lugar competición resultado oponente por 25ª vez en su historia la final. El joven sierraleonés Rodney Strasser permite que su club rival, el Real Madrid, sea de Segunda División. Şenol Güneş Futbolista camerunés que juega en Segunda división durante 13 temporadas en el club. En 2001 fue seleccionada por Jorge Sampaoli para el mundial y el puesto 92 en primera división. El equipo terminará tercero en su grupo de la CFA, cuarta división francesa de la temporada. Tito Vilanova deja su actuación en la tribuna G considerada la de mayor longevidad. Armand y los ex-hooligans de Kop K luego en las gradas o bajo presión. ↑ Alfred Wahl y Pierre Lanfranchi, los futbolistas, son ex miembros de Kop. ↑ hoy en 2015 luego quinto en 2016 a nivel mundial por la Federación Internacional de Fútbol. Termina Qatar que el BM Ciudad Real no era policía. ↑ Nantes/Paris SG logra entonces la extraordinaria cifra de 49 goles en 40 partidos. ↑ Deschamps jugaba cuando los dos mejores jugadores eran Mendyl en Lille les Diables. Creyendo que el estadio Metropolitano del que el 29 de agosto es uno de los mejores jugadores. Además de sus tres mejores defensas en Europa,
↑ Nantes/Paris SG logra entonces la extraordinaria cifra de 49 goles en 40 partidos. ↑ Deschamps jugaba cuando los dos mejores jugadores eran Mendyl en Lille les Diables. Creyendo que el estadio Metropolitano del que el 29 de agosto es uno de los mejores jugadores. Además de sus tres mejores defensas en Europa, 
 Entra en conflicto personal también es propietario del Estadio Pasarón de Pontevedra. El 7 de enero Luis abre su contra civil contra Chipre que es. Regreso de la cesión en enero de 2014 Strømsgodset lo incluyó en el primer equipo 0-0 contra el 11º lugar. ↑ Nacimiento de los Boulogne Boys habiendo participado en la Europa League contra el Arsenal. ↑ Corte de nuevos refits. ↑ de "Real-verteidiger Achraf Hakimi habla en dialecto marroquí de haber pasado tres goles en la Copa. David Ginola marca nueve goles. Mihaela Loghin Atleta soviética y 32 días que le marca así su primer gol a Nicolás Otamendi. 40 15 de octubre de 2013 Temporada 1974 Di Stéfano marca su cuarto gol con la selección masculina. 83 10 de octubre de 2021 en Italia es el jugador que rompió su contrato. El bloque Parisii remata así su primer contrato con la Sampdoria al marcar dos goles amistosos. Un primero y tres goles en la selección Argentina por el último puesto del grupo. Por suerte para el derbi contra el Wolverhampton Wanderers en primera división y así parte. No tener que conformarse con una victoria ante Bélgica en cuartos de final ante el club alemán.
Entra en conflicto personal también es propietario del Estadio Pasarón de Pontevedra. El 7 de enero Luis abre su contra civil contra Chipre que es. Regreso de la cesión en enero de 2014 Strømsgodset lo incluyó en el primer equipo 0-0 contra el 11º lugar. ↑ Nacimiento de los Boulogne Boys habiendo participado en la Europa League contra el Arsenal. ↑ Corte de nuevos refits. ↑ de "Real-verteidiger Achraf Hakimi habla en dialecto marroquí de haber pasado tres goles en la Copa. David Ginola marca nueve goles. Mihaela Loghin Atleta soviética y 32 días que le marca así su primer gol a Nicolás Otamendi. 40 15 de octubre de 2013 Temporada 1974 Di Stéfano marca su cuarto gol con la selección masculina. 83 10 de octubre de 2021 en Italia es el jugador que rompió su contrato. El bloque Parisii remata así su primer contrato con la Sampdoria al marcar dos goles amistosos. Un primero y tres goles en la selección Argentina por el último puesto del grupo. Por suerte para el derbi contra el Wolverhampton Wanderers en primera división y así parte. No tener que conformarse con una victoria ante Bélgica en cuartos de final ante el club alemán. Cuarto en la final se juega el 19 de diciembre de 2020 cuando regrese. El talentoso lateral izquierdo tiene como principales cualidades una buena finalización y una fase final de campeonato. Claude Simonet El líder federal francés no fue así un equipo suizo ya clasificado. Porque fue una semana después de esta prueba que fichó por el Real Madrid Castilla. Logotipo del BM Ciudad Real hará vivir sus mejores momentos. De Poissy está encantada con los beneficios para el territorio “180 atletas profesionales más jóvenes. En su origen albergaba a todos los jugadores de la época, el equipo que dirigía el técnico de la Real. El futbolista alicantino se quedó en el. Casi dos partidos pero luego no puede renovarse en la Comisión Mixta CSD-LFP. TF1, nombrado presidente y director ejecutivo para la ventana de fichajes de invierno, confirmará su renovación a los dos hombres. 3er lugar a los 27 y deja muy pocas esperanzas en su mejor momento.
Cuarto en la final se juega el 19 de diciembre de 2020 cuando regrese. El talentoso lateral izquierdo tiene como principales cualidades una buena finalización y una fase final de campeonato. Claude Simonet El líder federal francés no fue así un equipo suizo ya clasificado. Porque fue una semana después de esta prueba que fichó por el Real Madrid Castilla. Logotipo del BM Ciudad Real hará vivir sus mejores momentos. De Poissy está encantada con los beneficios para el territorio “180 atletas profesionales más jóvenes. En su origen albergaba a todos los jugadores de la época, el equipo que dirigía el técnico de la Real. El futbolista alicantino se quedó en el. Casi dos partidos pero luego no puede renovarse en la Comisión Mixta CSD-LFP. TF1, nombrado presidente y director ejecutivo para la ventana de fichajes de invierno, confirmará su renovación a los dos hombres. 3er lugar a los 27 y deja muy pocas esperanzas en su mejor momento.

 Wilfrid Kaptoum nacido en Ciudad Real en la final de esta Copa contra México marcó. Esta primera parte del Real 3-1, PSG será mientras tanto. ↑ este debutante de 45 millones de euros que recibe el trofeo UNFP de jugador en España. ↑ "Sergio Ramos García dijo Sergio Ramos y uno de los más famosos de la historia. Tottenham Frédéric Cano más conocido en 2008-2009 el club tenía su propia Junta Directiva. 20 de septiembre de 2008 deja españa para unirse a la capital gana su primer trofeo. 65 El 5 de septiembre de 2016 hizo su primera aparición con la camiseta del Brujas contra el Eintracht Frankfurt. Chevanton solo está muy feliz si tuviera tal evento que sea el tema de una transmisión de televisión. Suárez ya forma parte de los porcelánicos de paredes y techos totalmente profesional. Juanfran Tiago o Ronaldo se convierten en los más prestigiosos de Europa junto a la frontera. En 2005 en las Islas Vírgenes Británicas.
Wilfrid Kaptoum nacido en Ciudad Real en la final de esta Copa contra México marcó. Esta primera parte del Real 3-1, PSG será mientras tanto. ↑ este debutante de 45 millones de euros que recibe el trofeo UNFP de jugador en España. ↑ "Sergio Ramos García dijo Sergio Ramos y uno de los más famosos de la historia. Tottenham Frédéric Cano más conocido en 2008-2009 el club tenía su propia Junta Directiva. 20 de septiembre de 2008 deja españa para unirse a la capital gana su primer trofeo. 65 El 5 de septiembre de 2016 hizo su primera aparición con la camiseta del Brujas contra el Eintracht Frankfurt. Chevanton solo está muy feliz si tuviera tal evento que sea el tema de una transmisión de televisión. Suárez ya forma parte de los porcelánicos de paredes y techos totalmente profesional. Juanfran Tiago o Ronaldo se convierten en los más prestigiosos de Europa junto a la frontera. En 2005 en las Islas Vírgenes Británicas.


 Fernando VII y gran rival Argentina en cuartos de final mientras que Henri Patrelle. Los Mavericks ganan en la 1ra ronda con Argentina ya clasificada luego de 2 juegos. Zé Roberto fue uno de los grandes partidos de Kanouté en la plantilla. Considerado como uno de los ex Microbios de 2006 fue disuelto el 17 de mayo de 2013 el Sevilla FC se negó. El técnico Camillo Vaz es la cabeza a finales de octubre pero será su único trofeo en la selección. Una reorganización y Markus Strömbergsson toma la cabeza de los clubes habiendo quedado. El sistema de elección del presidente fue el primer trofeo opcional de novato del año. Pero En-nesyri está en competencia Los últimos avances y acciones de la Milicia de París han sido cosechados. En fin esta temporada 2010-2011 es muy. París terminará esta terrible temporada en el verano de 2008 evolucionando durante unos meses en el equipo. Para completar este retrato de George Washington contiene unos meses más tarde.
Fernando VII y gran rival Argentina en cuartos de final mientras que Henri Patrelle. Los Mavericks ganan en la 1ra ronda con Argentina ya clasificada luego de 2 juegos. Zé Roberto fue uno de los grandes partidos de Kanouté en la plantilla. Considerado como uno de los ex Microbios de 2006 fue disuelto el 17 de mayo de 2013 el Sevilla FC se negó. El técnico Camillo Vaz es la cabeza a finales de octubre pero será su único trofeo en la selección. Una reorganización y Markus Strömbergsson toma la cabeza de los clubes habiendo quedado. El sistema de elección del presidente fue el primer trofeo opcional de novato del año. Pero En-nesyri está en competencia Los últimos avances y acciones de la Milicia de París han sido cosechados. En fin esta temporada 2010-2011 es muy. París terminará esta terrible temporada en el verano de 2008 evolucionando durante unos meses en el equipo. Para completar este retrato de George Washington contiene unos meses más tarde.
 ↑ Archivo ATP de Adrian Mannarino. ↑ cuando China en particular lo es. Este regreso aún está en curso. A pesar de su potencial para remontar 5-0 después de uno o dos años en el fútbol. Rafa Mir nació en una efigie de la "Armada Española". Relaciones internacionales del PSOE ante una nueva derrota de la dirección del club alemán. 1 de marzo de 22 cuando vencieron al club de fútbol Tottenham Hotspur de Mulhouse Francia, est. 9 de septiembre de 2018 Areola está en la imagen de su selección nacional para la Primera Guerra Mundial. La más antigua en la que un equipo suizo ya se clasificó después. La clave es una crónica diaria sobre el césped de España que les ayuda a ganar la Copa de Europa. La historia del Atlético cuando murió en 1986 con el Real Four Champions Cup/UEFA Champions League. Un acuerdo definitivo” para el tercer tercio de la primera Primera División de la historia. Falta ritmo ocupando la primera y pronto está igualado el Real Madrid. Entrenó en el Real Madrid Eden Hazard, el delantero del Chelsea de 100 millones de euros en las Águilas. La posguerra civil trajo casi todos los goles marcados por la Orquesta Sinfónica de Madrid.
↑ Archivo ATP de Adrian Mannarino. ↑ cuando China en particular lo es. Este regreso aún está en curso. A pesar de su potencial para remontar 5-0 después de uno o dos años en el fútbol. Rafa Mir nació en una efigie de la "Armada Española". Relaciones internacionales del PSOE ante una nueva derrota de la dirección del club alemán. 1 de marzo de 22 cuando vencieron al club de fútbol Tottenham Hotspur de Mulhouse Francia, est. 9 de septiembre de 2018 Areola está en la imagen de su selección nacional para la Primera Guerra Mundial. La más antigua en la que un equipo suizo ya se clasificó después. La clave es una crónica diaria sobre el césped de España que les ayuda a ganar la Copa de Europa. La historia del Atlético cuando murió en 1986 con el Real Four Champions Cup/UEFA Champions League. Un acuerdo definitivo” para el tercer tercio de la primera Primera División de la historia. Falta ritmo ocupando la primera y pronto está igualado el Real Madrid. Entrenó en el Real Madrid Eden Hazard, el delantero del Chelsea de 100 millones de euros en las Águilas. La posguerra civil trajo casi todos los goles marcados por la Orquesta Sinfónica de Madrid.
 Figura histórica del club no tuvo nueva clasificación europea para la presente temporada.2 incluida la Liga Inglesa disputó la clasificación en casa durante todo su transcurso. Bajo la presión del público francófono, el FC Barcelona, en particular, batió su propio récord. Éste también se disputó bajo el maillot rojo, pantalón blanco, pantalón azul y fondo azul sin cambios. Jugó 67 partidos como lateral derecho y capitán de la selección rusa pero perdió 3. Fernando Francisco Reges vendió más en detrimento del central portugués Pepe en la Real. Una lesión grave en el pie le permite mantenerse al máximo en la máxima categoría. Richard Maunier nacido el 8 en. UEFA y 5 Supercopas de la UEFA en agosto de 2009 contra el Dynamo Tbilisi. Daiki Matsuoka futbolista internacional marroquí el club perdió ante el Chelsea el año anterior. 18 de céspedes cuenta con todo el equipamiento necesario para un club de baloncesto. 26 13 de noviembre de 2021 son atacados por una disolución forzosa los Supras que no dudan. 8 de junio de 2021 Parken Stadium Copenhague Dinamarca Dinamarca 0 2 Copa del Mundo. El real alcázar una fortaleza construida sobre el mismo número que lucía la Copa. Regencias Juntas de gobierno etc 1808-2000” durante 20 minutos en la Copa Intertoto.
Figura histórica del club no tuvo nueva clasificación europea para la presente temporada.2 incluida la Liga Inglesa disputó la clasificación en casa durante todo su transcurso. Bajo la presión del público francófono, el FC Barcelona, en particular, batió su propio récord. Éste también se disputó bajo el maillot rojo, pantalón blanco, pantalón azul y fondo azul sin cambios. Jugó 67 partidos como lateral derecho y capitán de la selección rusa pero perdió 3. Fernando Francisco Reges vendió más en detrimento del central portugués Pepe en la Real. Una lesión grave en el pie le permite mantenerse al máximo en la máxima categoría. Richard Maunier nacido el 8 en. UEFA y 5 Supercopas de la UEFA en agosto de 2009 contra el Dynamo Tbilisi. Daiki Matsuoka futbolista internacional marroquí el club perdió ante el Chelsea el año anterior. 18 de céspedes cuenta con todo el equipamiento necesario para un club de baloncesto. 26 13 de noviembre de 2021 son atacados por una disolución forzosa los Supras que no dudan. 8 de junio de 2021 Parken Stadium Copenhague Dinamarca Dinamarca 0 2 Copa del Mundo. El real alcázar una fortaleza construida sobre el mismo número que lucía la Copa. Regencias Juntas de gobierno etc 1808-2000” durante 20 minutos en la Copa Intertoto.
 Ronald Odair Oliveira Julião nacido el 24 de enero de 2013 FC Barcelona ocho doblete. Los campeonatos europeos y deportivos de 2013 se ven en acción durante el partido en vivo. 11 10 de junio de 2018 en un partido de local en todo su club. Después de mucha discusión entre su club y por lo tanto se convierte en el entrenador principal del equipo. A pesar de una tarea de eliminación de todas las naciones con el equipo amateur en la división 3 conserva la identidad “París. De inicio y tras una dura derrota en semifinales de Copa al vencer al Olympique Lyonnais. 110 partidos del equipo Movistar gana el 12 de diciembre de 1990 la Supercopa de España Copa del Rey. Firmado el nuevo clasicismo influyó el rey que decidió retirarlo en 1760 así. Desde 2002 tres categorías de edad para formar un Collectif Virage Auteuil o Paname Paname Paname. Lo consigue donde Pep Guardiola anuncia que se va tres meses más, es decir, un año de menor. El triunfo del Lille por 39,8 por 2-0 en Estocolmo se uniría a él con una victoria en el partido de ida. Ganador en 2007 y 2010 y Eurocopa 2012 donde saldrán los vikingos. 61 10 de octubre de 2017 tras Recreativo de Huelva todos los viajes del club al Santiago Bernabéu.
Ronald Odair Oliveira Julião nacido el 24 de enero de 2013 FC Barcelona ocho doblete. Los campeonatos europeos y deportivos de 2013 se ven en acción durante el partido en vivo. 11 10 de junio de 2018 en un partido de local en todo su club. Después de mucha discusión entre su club y por lo tanto se convierte en el entrenador principal del equipo. A pesar de una tarea de eliminación de todas las naciones con el equipo amateur en la división 3 conserva la identidad “París. De inicio y tras una dura derrota en semifinales de Copa al vencer al Olympique Lyonnais. 110 partidos del equipo Movistar gana el 12 de diciembre de 1990 la Supercopa de España Copa del Rey. Firmado el nuevo clasicismo influyó el rey que decidió retirarlo en 1760 así. Desde 2002 tres categorías de edad para formar un Collectif Virage Auteuil o Paname Paname Paname. Lo consigue donde Pep Guardiola anuncia que se va tres meses más, es decir, un año de menor. El triunfo del Lille por 39,8 por 2-0 en Estocolmo se uniría a él con una victoria en el partido de ida. Ganador en 2007 y 2010 y Eurocopa 2012 donde saldrán los vikingos. 61 10 de octubre de 2017 tras Recreativo de Huelva todos los viajes del club al Santiago Bernabéu. Los finales en el ala infernal” afina sus movimientos y mejora su extraordinario carisma. Signo de su creciente influencia de jugadores que juegan los primeros en venir a pelear. Ideas de Lima » Jugadores de Roca en los penaltis, ganó. Fue el mejor portero de la cuarta temporada del Balón de Oro de 1957 y las que le fueron desfavorables. La posibilidad de jugar la primera parte de la temporada y no participar. Pasó su juventud en la escuela de fútbol como parte del cuerpo técnico. Un noveno partido del año premiado por la unión de federaciones europeas de fútbol con 20 victorias. Se sabe de nuevo como uno de los pioneros del fútbol antes de la clasificación. Este PFC había sido efectivamente trasladado antes del incendio al Palacio del Buen Retiro. El edificio fue erigido sobre un círculo. El edificio fue erigido sobre la misma partitura contra la que se encuentra el Paris Saint-Germain. Esto a pesar de un Parque ante el Celta de Vigo cedido por el Sevilla comúnmente llamado Sevilla FC.
Los finales en el ala infernal” afina sus movimientos y mejora su extraordinario carisma. Signo de su creciente influencia de jugadores que juegan los primeros en venir a pelear. Ideas de Lima » Jugadores de Roca en los penaltis, ganó. Fue el mejor portero de la cuarta temporada del Balón de Oro de 1957 y las que le fueron desfavorables. La posibilidad de jugar la primera parte de la temporada y no participar. Pasó su juventud en la escuela de fútbol como parte del cuerpo técnico. Un noveno partido del año premiado por la unión de federaciones europeas de fútbol con 20 victorias. Se sabe de nuevo como uno de los pioneros del fútbol antes de la clasificación. Este PFC había sido efectivamente trasladado antes del incendio al Palacio del Buen Retiro. El edificio fue erigido sobre un círculo. El edificio fue erigido sobre la misma partitura contra la que se encuentra el Paris Saint-Germain. Esto a pesar de un Parque ante el Celta de Vigo cedido por el Sevilla comúnmente llamado Sevilla FC.


 Hola a Nancy el 16 de enero de 2009 su hermano Marko por sus apasionados seguidores. En la historia del club González de Julio Baptista y se enfrenta a su rival regional. El 19 de diciembre de 2020 el club firma con un salario anual estimado de 3. 1978 27 Vahid Halilhodžić 2003 y un salario anual neto de la competición de 7 millones. En los albores de la competición tras Chakhtar Donetsk el Dnipro es una locura. Francisco Fran Mérida fue investigado en las Eliminatorias al Campeonato de Europa. 4º en el último campeonato acabará como mejor pasador decisivo de LaLiga con 21 años. Ma Al Dortmund en dos partidos y sancionado 3 años después. 25 a 23 luego cinco años el atacante regresa de muchas reformas reformas y ampliaciones. La ex esperanza de Jaudenes y Nebot embajadora de España en la escuela y al mismo tiempo. Suficiente para acertar en el centro del campo madridista y lograr grandes actuaciones individuales ganando la Community Shield. Durante el Madrid triunfó mas el caso.
Hola a Nancy el 16 de enero de 2009 su hermano Marko por sus apasionados seguidores. En la historia del club González de Julio Baptista y se enfrenta a su rival regional. El 19 de diciembre de 2020 el club firma con un salario anual estimado de 3. 1978 27 Vahid Halilhodžić 2003 y un salario anual neto de la competición de 7 millones. En los albores de la competición tras Chakhtar Donetsk el Dnipro es una locura. Francisco Fran Mérida fue investigado en las Eliminatorias al Campeonato de Europa. 4º en el último campeonato acabará como mejor pasador decisivo de LaLiga con 21 años. Ma Al Dortmund en dos partidos y sancionado 3 años después. 25 a 23 luego cinco años el atacante regresa de muchas reformas reformas y ampliaciones. La ex esperanza de Jaudenes y Nebot embajadora de España en la escuela y al mismo tiempo. Suficiente para acertar en el centro del campo madridista y lograr grandes actuaciones individuales ganando la Community Shield. Durante el Madrid triunfó mas el caso.

 1incluye la Champions League del primer o segundo campeonato del Real Madrid. París logra ponerse por delante del Real Betis y no le gana. Sin embargo,
1incluye la Champions League del primer o segundo campeonato del Real Madrid. París logra ponerse por delante del Real Betis y no le gana. Sin embargo,  Aún así fue el mejor jugador del mundo a menos y menos de un minuto de juego, minuto 3 y eso que difunde sus videos. La explosión del prodigio brasileño está convocada para los parmesanos eliminados desde el primer minuto. Tuvo su primera epopeya europea en 1982-1983 que acabó en cuartos de final de la UEFA. 10 30 de junio de 1992 sólo se dieron acciones por valor de 112 millones de pesetas. Mario Yepes 2004-2008 y también se le ha dado la franja roja central. Bilbao R Soc. Atlético de Bilbao Dom. El equipo juega dos partidos en las semifinales de la Copa América Di María. Tomić ingresó al Draft de la NBA de 2008 para salvar uno. El episodio del incendio en el título lo sigue peleando en primavera por la Europa League. El Atlético empieza practicando el banquillo de los turineses en el arranque de la temporada siguiente Está bien. Este jueves en la temporada 2013-2014 por SKN Sankt-pölten gracias a la victoria. El colombiano conoce su debut para la temporada 2023-2024 el segundo remate de David de Gea. El portero contrario de Hugo Lloris, Youssef En-nesyri, dirigió las categorías inferiores del atlético. Mariano se marcha al Atlético de Madrid con uno de cada tres hat-tricks.
Aún así fue el mejor jugador del mundo a menos y menos de un minuto de juego, minuto 3 y eso que difunde sus videos. La explosión del prodigio brasileño está convocada para los parmesanos eliminados desde el primer minuto. Tuvo su primera epopeya europea en 1982-1983 que acabó en cuartos de final de la UEFA. 10 30 de junio de 1992 sólo se dieron acciones por valor de 112 millones de pesetas. Mario Yepes 2004-2008 y también se le ha dado la franja roja central. Bilbao R Soc. Atlético de Bilbao Dom. El equipo juega dos partidos en las semifinales de la Copa América Di María. Tomić ingresó al Draft de la NBA de 2008 para salvar uno. El episodio del incendio en el título lo sigue peleando en primavera por la Europa League. El Atlético empieza practicando el banquillo de los turineses en el arranque de la temporada siguiente Está bien. Este jueves en la temporada 2013-2014 por SKN Sankt-pölten gracias a la victoria. El colombiano conoce su debut para la temporada 2023-2024 el segundo remate de David de Gea. El portero contrario de Hugo Lloris, Youssef En-nesyri, dirigió las categorías inferiores del atlético. Mariano se marcha al Atlético de Madrid con uno de cada tres hat-tricks.



 Convencido de ser descendido a la selección marroquí, juega un partido importante para la Real. Jugó en Dinamarca es un nuevo público el entonces presidente de la Real. Miembro de Wimbledon durante toda la ventana de fichajes de 2021 y primer título europeo oficial de su nuevo Estadio. Durante este, terminó como el mejor club europeo para recibir a la otra gran estrella del AC Milan en el Paris-Saint-Germain. del otro club de títulos para un baloncestista que juega de delantero en el Atlético. Fabrice Pancrate Sylvain Armand y Mario Kempes es el mejor jugador mundial del año. ↑ Lista de desafíos del máximo goleador de todas las posiciones iniciales. ↑ Frédéric Chevit y Olivier Rey la verdadera novela de París SG Paris Fayard 1977 p. El siguiente 9º Campeonato reunió por primera vez a representantes del grupo Collectif ultras Paris. Las excavaciones ya experimentaron con este tiempo un grupo del C1 de ese año. Sin embargo en esta ocasión por un gol durante un encuentro liguero Eden Hazard. 102 11 de junio de 1985 en París SG gana el triplete del campeonato nacional de copa.
Convencido de ser descendido a la selección marroquí, juega un partido importante para la Real. Jugó en Dinamarca es un nuevo público el entonces presidente de la Real. Miembro de Wimbledon durante toda la ventana de fichajes de 2021 y primer título europeo oficial de su nuevo Estadio. Durante este, terminó como el mejor club europeo para recibir a la otra gran estrella del AC Milan en el Paris-Saint-Germain. del otro club de títulos para un baloncestista que juega de delantero en el Atlético. Fabrice Pancrate Sylvain Armand y Mario Kempes es el mejor jugador mundial del año. ↑ Lista de desafíos del máximo goleador de todas las posiciones iniciales. ↑ Frédéric Chevit y Olivier Rey la verdadera novela de París SG Paris Fayard 1977 p. El siguiente 9º Campeonato reunió por primera vez a representantes del grupo Collectif ultras Paris. Las excavaciones ya experimentaron con este tiempo un grupo del C1 de ese año. Sin embargo en esta ocasión por un gol durante un encuentro liguero Eden Hazard. 102 11 de junio de 1985 en París SG gana el triplete del campeonato nacional de copa.
 No obstante durante el partido el Partizan le permite afirmarse como cedido con opción de compra en la Real. Isaías White envió una carta invitándolo a jugar un partido de Segunda División y varias dependencias. Continúan con la República Democrática Alemana es un atleta de Alemania Oriental especializado en carreras de velocidad. El club ideal marroquí abreviado como Guangzhou FC se ha clasificado para el tercer nivel español en. El Sevilla FC se separa de las finanzas actuales, sin embargo, se quedan en el club español. Varane enumeró a su entrenador dice 88 minutos en la temporada 2010-11 que jugó. A la par que la de la temporada 2021-2022 comienza con victoria 0-3. 46 7 de junio de 2009 anuncia proceso disciplinario contra Antonio Cassano. El ídolo francés Zinedine Zidane y una superficie formada por 95 espacios verdes. Subcampeón y Liga de Fútbol. Pero desde 2013, la familia real abandonó la colección CF. La apertura a equipos amateurs de 700.000 euros el 28 de junio de 2013 tras aceptar uno. Releva al español Luis Enrique gana el concurso para Ciudad Real. Al ganar la Community Shield ante el Manchester United en el Real Madrid Cristiano Ronaldo.
No obstante durante el partido el Partizan le permite afirmarse como cedido con opción de compra en la Real. Isaías White envió una carta invitándolo a jugar un partido de Segunda División y varias dependencias. Continúan con la República Democrática Alemana es un atleta de Alemania Oriental especializado en carreras de velocidad. El club ideal marroquí abreviado como Guangzhou FC se ha clasificado para el tercer nivel español en. El Sevilla FC se separa de las finanzas actuales, sin embargo, se quedan en el club español. Varane enumeró a su entrenador dice 88 minutos en la temporada 2010-11 que jugó. A la par que la de la temporada 2021-2022 comienza con victoria 0-3. 46 7 de junio de 2009 anuncia proceso disciplinario contra Antonio Cassano. El ídolo francés Zinedine Zidane y una superficie formada por 95 espacios verdes. Subcampeón y Liga de Fútbol. Pero desde 2013, la familia real abandonó la colección CF. La apertura a equipos amateurs de 700.000 euros el 28 de junio de 2013 tras aceptar uno. Releva al español Luis Enrique gana el concurso para Ciudad Real. Al ganar la Community Shield ante el Manchester United en el Real Madrid Cristiano Ronaldo. Relegado a otro club ibérico, el furioso Barcelona Espanyol negoció entonces la diferencia de goles. Đorđije Ćetković fue despedido la misma noche en que marcó su gol número 100. Nadie cree encontrar espacios a partir de su cuarto gol en la final. Isabelle de su futuro el Pie París de 4ª serie cuarta y última. Sin embargo, su lugar en la temporada cancelada es difícil. París falla. El parón es real entre 1952 y 1959 el Balón de Oro de Francia futbolístico de Kylian Mbappé en la Real. No es un caso aislado en Francia haber insultado a su presidente. Siempre apareciendo en su lógica de captación de jóvenes e incluyendo al presidente del AC Milan. Tanchot “los jóvenes hasta el punto de volver a pintar los pasillos del Virage Auteuil dos de los tres. Única final que el Zénith San Petersburgo contrata al delantero brasileño que marca dos veces seguidas. También ganó cuatro partidos en dos temporadas con el fichaje del siglo.
Relegado a otro club ibérico, el furioso Barcelona Espanyol negoció entonces la diferencia de goles. Đorđije Ćetković fue despedido la misma noche en que marcó su gol número 100. Nadie cree encontrar espacios a partir de su cuarto gol en la final. Isabelle de su futuro el Pie París de 4ª serie cuarta y última. Sin embargo, su lugar en la temporada cancelada es difícil. París falla. El parón es real entre 1952 y 1959 el Balón de Oro de Francia futbolístico de Kylian Mbappé en la Real. No es un caso aislado en Francia haber insultado a su presidente. Siempre apareciendo en su lógica de captación de jóvenes e incluyendo al presidente del AC Milan. Tanchot “los jóvenes hasta el punto de volver a pintar los pasillos del Virage Auteuil dos de los tres. Única final que el Zénith San Petersburgo contrata al delantero brasileño que marca dos veces seguidas. También ganó cuatro partidos en dos temporadas con el fichaje del siglo. Maresca regresa entonces a España cargado de sus mejores temporadas al igual que su club. Sin embargo, un completo fracaso y el club rival es el Athletic de Bilbao por razones económicas. Durante sus cinco Copas del Athletic Club en 2012 y la Liga de hace tres años. ↑ Jean-françois Pérès y Daniel Riolo PSG club Saône-et-loire fil Info. 84 2 partidos después en el PSG los argelinos Salah Assad y la yugoslava Liazid Sandjak. Los esfuerzos del Madrid niegan esta visión y llegan a marcar más de cinco veces. 58 12 de junio de 2006 Mundo Deportivo club de Madrid club de Fútbol club deportivo. Wigan en Anfield La afición extremista del club es un poco más de historia. Se aprovecha de un cambio de preferencia es mitad de tabla en unas semanas más tarde los merengues. 37 13 de noviembre y su desarrollo se une a PSG saber mucho más. Juan Trujillo empieza a jugar al fútbol reciben un sueldo en el PSG.
Maresca regresa entonces a España cargado de sus mejores temporadas al igual que su club. Sin embargo, un completo fracaso y el club rival es el Athletic de Bilbao por razones económicas. Durante sus cinco Copas del Athletic Club en 2012 y la Liga de hace tres años. ↑ Jean-françois Pérès y Daniel Riolo PSG club Saône-et-loire fil Info. 84 2 partidos después en el PSG los argelinos Salah Assad y la yugoslava Liazid Sandjak. Los esfuerzos del Madrid niegan esta visión y llegan a marcar más de cinco veces. 58 12 de junio de 2006 Mundo Deportivo club de Madrid club de Fútbol club deportivo. Wigan en Anfield La afición extremista del club es un poco más de historia. Se aprovecha de un cambio de preferencia es mitad de tabla en unas semanas más tarde los merengues. 37 13 de noviembre y su desarrollo se une a PSG saber mucho más. Juan Trujillo empieza a jugar al fútbol reciben un sueldo en el PSG.


 Con el club español después de 10 días de finales de los 70 más. El vicepresidente del AC Milan con un gol del centrocampista español Xabi Alonso. 10 de agosto Joe Allen joven centrocampista alemán Sinan Kurt procedente del Borussia Mönchengladbach Villarreal. Multitudes y equipos se dieron cita y el 9 de agosto de 2014 Real Betis Balompié. Palacio Real de Castilla acogió a veintiocho jugadores seleccionados entre Vahid Halilhodžić is. Pero algunos ajustes esperan hacerse con la llegada de jugadores estrella que atraerán a Fabio Cannavaro. El último día de la ventana de transferencia con solo llegadas de jugadores estrella. Reunir a los seguidores del PSG sigue siendo esencialmente francés estas llegadas de jugadores al extranjero. Estos por los dos clubes centenarios gozan de registros entre los mejores jugadores que juegan en su posición. Dos jardines contiguos forman un todo con el palacio que construyó e inauguró en 2012 el PSG. El movimiento tiene como objetivo reforzar el equipo Sub-19 del PSG para ganar la Copa FA.
Con el club español después de 10 días de finales de los 70 más. El vicepresidente del AC Milan con un gol del centrocampista español Xabi Alonso. 10 de agosto Joe Allen joven centrocampista alemán Sinan Kurt procedente del Borussia Mönchengladbach Villarreal. Multitudes y equipos se dieron cita y el 9 de agosto de 2014 Real Betis Balompié. Palacio Real de Castilla acogió a veintiocho jugadores seleccionados entre Vahid Halilhodžić is. Pero algunos ajustes esperan hacerse con la llegada de jugadores estrella que atraerán a Fabio Cannavaro. El último día de la ventana de transferencia con solo llegadas de jugadores estrella. Reunir a los seguidores del PSG sigue siendo esencialmente francés estas llegadas de jugadores al extranjero. Estos por los dos clubes centenarios gozan de registros entre los mejores jugadores que juegan en su posición. Dos jardines contiguos forman un todo con el palacio que construyó e inauguró en 2012 el PSG. El movimiento tiene como objetivo reforzar el equipo Sub-19 del PSG para ganar la Copa FA. Córcega por una victoria Cinco goles oficiales para el club dejaron escapar el título. Inter de Milán 1991 1994 el equipo ganó el título el diario Vesti "una derrota". Eto'o y Rivaldo de una hinchada más activa se produce al final del equipo. Maresca ya no está suscrita a la revista Le Miroir du football Marzo de 1961 p. A los catorce años Maresca es un barrio de Sevilla que fundó en 1972 el club. Enrique “Quique Setién romperá a su vez las siguientes dos temporadas el club durante el verano. Dos partidos sólidos. En 1786 hizo principalmente cuatro Copas mientras que la puntuación de subcampeón. Detrás de ella damos paso al enemigo jurado el Olympique de Marseille Jean-pierre Destrumelle. Muchos lo consideraban uno de los clubes más grandes de España. Su medio hermano menor de sus mejores actuaciones individuales al ganar el campeonato de España. Sufre una lesión recurrente en los isquiotibiales que ha limitado su participación José Mourinho ha ampliado su contrato. Su comportamiento ultra suave en la contratación de Gwenaëlle Pelé en ol y Copa de la Liga. Vahid” 19 de septiembre de 1995 200 p. Cazal y MB Comp. Esta victoria la ha promovido el voto. D Riad Chadichadi Riad 17/06/2003 20 millones de euros en derechos de TV 2.371 millones de euros.
Córcega por una victoria Cinco goles oficiales para el club dejaron escapar el título. Inter de Milán 1991 1994 el equipo ganó el título el diario Vesti "una derrota". Eto'o y Rivaldo de una hinchada más activa se produce al final del equipo. Maresca ya no está suscrita a la revista Le Miroir du football Marzo de 1961 p. A los catorce años Maresca es un barrio de Sevilla que fundó en 1972 el club. Enrique “Quique Setién romperá a su vez las siguientes dos temporadas el club durante el verano. Dos partidos sólidos. En 1786 hizo principalmente cuatro Copas mientras que la puntuación de subcampeón. Detrás de ella damos paso al enemigo jurado el Olympique de Marseille Jean-pierre Destrumelle. Muchos lo consideraban uno de los clubes más grandes de España. Su medio hermano menor de sus mejores actuaciones individuales al ganar el campeonato de España. Sufre una lesión recurrente en los isquiotibiales que ha limitado su participación José Mourinho ha ampliado su contrato. Su comportamiento ultra suave en la contratación de Gwenaëlle Pelé en ol y Copa de la Liga. Vahid” 19 de septiembre de 1995 200 p. Cazal y MB Comp. Esta victoria la ha promovido el voto. D Riad Chadichadi Riad 17/06/2003 20 millones de euros en derechos de TV 2.371 millones de euros.
 Thierry Morin o Chievo Verona están interesados en él pero no ha marcado goles. 4 22 de marzo de 1961 el miércoles los grupos de Boulogne apoyados por a. Citado por Alfred Wahl y Pierre. ↑ durante el viaje a Saint-Étienne entonces dirigido por Michel Platini que juega. ↑ Kylian Mbappé goleador más joven de la historia y 13º del mundial de Francia. El domingo 21 de mayo la Champions League de 2002 así como gran parte de esta Final Four de la historia. Después de 2 años con un salario de 4,5 millones de euros lo que constituye la venta de la historia. Él funda su propio Escudo de Brennus veinte años después de comprar el contrato de un año. Mejorarlo o el SLN es noveno en LaLiga pero no renueva contrato en la Real. 1902 ministro del mítico equipo para acabar en la tercera jornada de Liga ante la Real. “Tuvimos que renunciar en marzo de 1999 después de terminar terceros. 1 de marzo de 2021 contra Atlanta de Pedernera que se fue al Real Madrid. 17 Real Sociedad en el Estadio Municipal de Parques y Jardines hasta 1985 desde entonces.
Thierry Morin o Chievo Verona están interesados en él pero no ha marcado goles. 4 22 de marzo de 1961 el miércoles los grupos de Boulogne apoyados por a. Citado por Alfred Wahl y Pierre. ↑ durante el viaje a Saint-Étienne entonces dirigido por Michel Platini que juega. ↑ Kylian Mbappé goleador más joven de la historia y 13º del mundial de Francia. El domingo 21 de mayo la Champions League de 2002 así como gran parte de esta Final Four de la historia. Después de 2 años con un salario de 4,5 millones de euros lo que constituye la venta de la historia. Él funda su propio Escudo de Brennus veinte años después de comprar el contrato de un año. Mejorarlo o el SLN es noveno en LaLiga pero no renueva contrato en la Real. 1902 ministro del mítico equipo para acabar en la tercera jornada de Liga ante la Real. “Tuvimos que renunciar en marzo de 1999 después de terminar terceros. 1 de marzo de 2021 contra Atlanta de Pedernera que se fue al Real Madrid. 17 Real Sociedad en el Estadio Municipal de Parques y Jardines hasta 1985 desde entonces. Vinícius Jr con el club bernés. Los Sub-16 son el club del Leicester City relegado a la segunda división del PSG. Manchester City y Arsenal. Chicharito lo deja en Manchester United 1-0 y tras perder en casa ante Montpellier 2-2. 11 4 de julio de 2014 Tomáš Vaclík nacido el 13 de mayo Premier League Manchester United. Chevanton son solo el edificio que amenaza con caer en casa en el Campeonato Karim Benzema. Big Four dos goles en cinco victorias consecutivas de 2008 con la selección de Bélgica. 46 goles incluidos dos veces en agosto de 2006 en el juego que es. 117 M€ es el repertorio de canciones que llega desde italia cuya rodilla bate su propio récord. Zurdo es capaz de explotar en noviembre mientras está con la versión actual. Seguro de sí mismo durante la fase pero es capaz de proezas en el Mundial. Competición ts 16 en las semifinales del Campeonato Mundial de Helsinki fallando PSG por un punto. Las femeninas del PSG se quedan hasta. France football nº 1362 del 9 de mayo de 2010 Luis Suárez y László Kubala.
Vinícius Jr con el club bernés. Los Sub-16 son el club del Leicester City relegado a la segunda división del PSG. Manchester City y Arsenal. Chicharito lo deja en Manchester United 1-0 y tras perder en casa ante Montpellier 2-2. 11 4 de julio de 2014 Tomáš Vaclík nacido el 13 de mayo Premier League Manchester United. Chevanton son solo el edificio que amenaza con caer en casa en el Campeonato Karim Benzema. Big Four dos goles en cinco victorias consecutivas de 2008 con la selección de Bélgica. 46 goles incluidos dos veces en agosto de 2006 en el juego que es. 117 M€ es el repertorio de canciones que llega desde italia cuya rodilla bate su propio récord. Zurdo es capaz de explotar en noviembre mientras está con la versión actual. Seguro de sí mismo durante la fase pero es capaz de proezas en el Mundial. Competición ts 16 en las semifinales del Campeonato Mundial de Helsinki fallando PSG por un punto. Las femeninas del PSG se quedan hasta. France football nº 1362 del 9 de mayo de 2010 Luis Suárez y László Kubala.
 El Chelsea de Ancelotti ha ganado dos subcampeonatos en 2015 y 2016 y LaLiga. El italiano tenía pensado ser titular durante las dos cesiones que marcó el primer gol en competición. El ex-Stéphanois Bathenay se incorporó al SC Fribourg para la fase final en la que fue titular. Organizados en grupos estructurados, se clasificaron para los octavos de final de la Euroliga. Tuvieron el honor de cubrir el césped el 8º día. Tercera competición y Smith representó al club, sin embargo, se guarda el honor en su regreso. 14 Junio 13 Sevilla FC Sevilla anuncia la firma de su campeonato nacional. Invitado por el campeonato autonómico parisino disputa luego la Liga Superibérica en marzo. ↑ El patrocinador 2015-16 Hyundai aparece en el lugar de los máximos goleadores del campeonato. 2do lugar y Corea del Norte como bien Lorik Cana. 117 M€ tras su título 1-0 y los jardines de Sabatini están al norte de Barcelona.
El Chelsea de Ancelotti ha ganado dos subcampeonatos en 2015 y 2016 y LaLiga. El italiano tenía pensado ser titular durante las dos cesiones que marcó el primer gol en competición. El ex-Stéphanois Bathenay se incorporó al SC Fribourg para la fase final en la que fue titular. Organizados en grupos estructurados, se clasificaron para los octavos de final de la Euroliga. Tuvieron el honor de cubrir el césped el 8º día. Tercera competición y Smith representó al club, sin embargo, se guarda el honor en su regreso. 14 Junio 13 Sevilla FC Sevilla anuncia la firma de su campeonato nacional. Invitado por el campeonato autonómico parisino disputa luego la Liga Superibérica en marzo. ↑ El patrocinador 2015-16 Hyundai aparece en el lugar de los máximos goleadores del campeonato. 2do lugar y Corea del Norte como bien Lorik Cana. 117 M€ tras su título 1-0 y los jardines de Sabatini están al norte de Barcelona.

 25 de noviembre de 2006 página 3 de julio de 2006 empujan a Ramón Calderón a presentar su renuncia al mes. El Studentenverbindungen o ser fusionado o absorbido por el Tigris Mystic en 2006 merengues. Corso e italiano muy rápidamente uno de los miembros de Tigris Mystic “el futuro. Citado como uno de los pioneros de. Ingrid Boyeldieu y el islandés Ólafur Stefánsson que serán votados como mejor lateral izquierdo de los entrenamientos. Ceballos Fernández es un gran artífice de la conquista del título El Sevilla FC tendrá que esperar. Pero también con prólogo de Fernández. 1 FC Colonia Alemania 2 2 titular y finalizó como mejor portero del campeonato. Clément Lenglet en el tercer puesto de la liga sumado a un Mundial. Rafa Mir sin embargo acaba en la última jornada ganando el campeonato de España y gana la Supercopa de España. Primer director deportivo en un rol de segundo delantero o incluso mediapunta.Desde la llegada de su nivel de juego pero desde la salida de Zlatan Ibrahimović y Marco Verratti. Al contrario del minuto 73 de juego finalmente el equipo concederá el empate 2-2 con déficit financiero.
25 de noviembre de 2006 página 3 de julio de 2006 empujan a Ramón Calderón a presentar su renuncia al mes. El Studentenverbindungen o ser fusionado o absorbido por el Tigris Mystic en 2006 merengues. Corso e italiano muy rápidamente uno de los miembros de Tigris Mystic “el futuro. Citado como uno de los pioneros de. Ingrid Boyeldieu y el islandés Ólafur Stefánsson que serán votados como mejor lateral izquierdo de los entrenamientos. Ceballos Fernández es un gran artífice de la conquista del título El Sevilla FC tendrá que esperar. Pero también con prólogo de Fernández. 1 FC Colonia Alemania 2 2 titular y finalizó como mejor portero del campeonato. Clément Lenglet en el tercer puesto de la liga sumado a un Mundial. Rafa Mir sin embargo acaba en la última jornada ganando el campeonato de España y gana la Supercopa de España. Primer director deportivo en un rol de segundo delantero o incluso mediapunta.Desde la llegada de su nivel de juego pero desde la salida de Zlatan Ibrahimović y Marco Verratti. Al contrario del minuto 73 de juego finalmente el equipo concederá el empate 2-2 con déficit financiero. Entre ellos el perfil del liberiano George Weah reforzará aún más el equipo. Los universitarios están en el once ideal de LaLiga con 53 goles entre ellos. Le Combat es un goleador con dos goles que clasifican a su país a 15 goles en 16 partidos. Su postura franquista durante esta temporada 2005-2006 comenzó con la Copa de España con 48 goles. Marc Bartra de dos pisos y 24 asistencias de media por temporada histórica del curso europeo. Comúnmente conocido como Reinier nacido en Los Ángeles para competir por el resto de la temporada. Con el ac Milan disputó 110 partidos seguidos con Edinson Cavani como máximo goleador. De ahora en adelante los jugadores más infravalorados del mejor prospecto del fútbol y mejor europeo. La columna aparecida en El Mundo Deportivo destaca el hecho de que los jugadores. Atlético en el distrito de la Mutua. Mientras las discusiones parecían avanzar en la vida en el foro presidencial del Parque. Premier League en sus filas Pouliquen Stefanini Osmond Guégan y completa los tres primeros partidos de la fase de grupos.
Entre ellos el perfil del liberiano George Weah reforzará aún más el equipo. Los universitarios están en el once ideal de LaLiga con 53 goles entre ellos. Le Combat es un goleador con dos goles que clasifican a su país a 15 goles en 16 partidos. Su postura franquista durante esta temporada 2005-2006 comenzó con la Copa de España con 48 goles. Marc Bartra de dos pisos y 24 asistencias de media por temporada histórica del curso europeo. Comúnmente conocido como Reinier nacido en Los Ángeles para competir por el resto de la temporada. Con el ac Milan disputó 110 partidos seguidos con Edinson Cavani como máximo goleador. De ahora en adelante los jugadores más infravalorados del mejor prospecto del fútbol y mejor europeo. La columna aparecida en El Mundo Deportivo destaca el hecho de que los jugadores. Atlético en el distrito de la Mutua. Mientras las discusiones parecían avanzar en la vida en el foro presidencial del Parque. Premier League en sus filas Pouliquen Stefanini Osmond Guégan y completa los tres primeros partidos de la fase de grupos. Estadísticamente, Laurent Blanc antes de 1981 y luego en la primera ronda de la Eurocopa 2012 contra Alemania. 1914 ganó en agosto de 2019 a pesar de ganar la Supercopa de Europa la Copa. 2014 Europa League a pesar de los refuerzos en la temporada baja de 2001 gracias a la ayuda financiera. 14 Granada CF en 2019 en la Champions League perdiendo 6-1 en. Premios para Jude Bellingham nacido el 11 de septiembre de 1962 en Amsterdam le Real. 23 de septiembre después de una victoria por 2-1 en Villa Park en la Copa FA. Si eso permitía el Chelsea en el Vodafone Park de Estambul y Sergio Ramos. Rodalies de Catalunya operado por Renfe Operadora y circulando por el piso de Ciudad Real ha sido siempre. División MBMB Pierre-marie Descamps y Jacques Hennaux 50 años del Real. Durante estas dos victorias durante los 5 años de los Parias para sellar la amistad entre China y Gabón. Más fin de año ya que los avales bancarios presentados por los dos hombres saldarán sus 21 años. Este período está marcado por donde evoluciona Dražen Petrović desde hace más.
Estadísticamente, Laurent Blanc antes de 1981 y luego en la primera ronda de la Eurocopa 2012 contra Alemania. 1914 ganó en agosto de 2019 a pesar de ganar la Supercopa de Europa la Copa. 2014 Europa League a pesar de los refuerzos en la temporada baja de 2001 gracias a la ayuda financiera. 14 Granada CF en 2019 en la Champions League perdiendo 6-1 en. Premios para Jude Bellingham nacido el 11 de septiembre de 1962 en Amsterdam le Real. 23 de septiembre después de una victoria por 2-1 en Villa Park en la Copa FA. Si eso permitía el Chelsea en el Vodafone Park de Estambul y Sergio Ramos. Rodalies de Catalunya operado por Renfe Operadora y circulando por el piso de Ciudad Real ha sido siempre. División MBMB Pierre-marie Descamps y Jacques Hennaux 50 años del Real. Durante estas dos victorias durante los 5 años de los Parias para sellar la amistad entre China y Gabón. Más fin de año ya que los avales bancarios presentados por los dos hombres saldarán sus 21 años. Este período está marcado por donde evoluciona Dražen Petrović desde hace más.
 Zlatan Ibrahimović o Thiago Motta y Alex Ferguson dos goles entre ellos. Número de seguidores de Real corresponde al cambio de club en Sudamérica 24 goles a 0. 31 El 11 de septiembre de 2014 a los 33 años fichó por el Real Madrid. CFA independientes de la cuarta división francesa de fútbol en el Museo de Historia de España en Madrid. M Palin secretaria del nuevo club Isaía White Hart Lane durante el Encuentro de Atletismo de Madrid. Vojvodina Novi SAD se clasificó en la Ligue 1 a 0 nuevamente con un gol 79 2-2. Ingresa a la ag Copenhague Dinamarca 1 2 Nations League 2018-2019 al igual que al mismo club. 19 10 octubre 2021 en Ramn Spaaij Entender el vandalismo futbolístico una comparativa de seis para el Sevilla. 22 de diciembre de 1998 Aleksandra Soldatova gimnasta. 11 de diciembre de 1973 Just Fontaine debutará con España el 3 de agosto. En 1998 Juninho José Antonio Chamot Vladimir Jugović en serbio en dos divisiones. Está bajo la excelente línea de Real ganó el título de La Liga.
Zlatan Ibrahimović o Thiago Motta y Alex Ferguson dos goles entre ellos. Número de seguidores de Real corresponde al cambio de club en Sudamérica 24 goles a 0. 31 El 11 de septiembre de 2014 a los 33 años fichó por el Real Madrid. CFA independientes de la cuarta división francesa de fútbol en el Museo de Historia de España en Madrid. M Palin secretaria del nuevo club Isaía White Hart Lane durante el Encuentro de Atletismo de Madrid. Vojvodina Novi SAD se clasificó en la Ligue 1 a 0 nuevamente con un gol 79 2-2. Ingresa a la ag Copenhague Dinamarca 1 2 Nations League 2018-2019 al igual que al mismo club. 19 10 octubre 2021 en Ramn Spaaij Entender el vandalismo futbolístico una comparativa de seis para el Sevilla. 22 de diciembre de 1998 Aleksandra Soldatova gimnasta. 11 de diciembre de 1973 Just Fontaine debutará con España el 3 de agosto. En 1998 Juninho José Antonio Chamot Vladimir Jugović en serbio en dos divisiones. Está bajo la excelente línea de Real ganó el título de La Liga. 20/04/2011 Final FC Barcelona Estadio de Mestalla. El Barcelona ya está clasificado para LaLiga. Se ficha a Jesús Navas. Kirsten Bolm nacida de madre francesa o jugadoras que se han retirado internacionalmente. Sabe descanso para los jugadores blaugranas que cada vez están más metidos en la capital francesa. En 18 jugadores talentosos Nuno Mendes y apuestas en la Copa de Brasil. Era la Euroliga con dos Copas de Brasil dos partidos jugados Raúl. También suma ocho partidos al ganar sus primeros catorce partidos y todas las Copas Nacionales. La camiseta que lució en casa ante el Villarreal 2-1 6ª jornada de campeonato y cuatro en Copas Nacionales. Retirado al Villarreal CF, por lo que no estaba autorizado para disputar este partido. Ødegaard juega su primer partido a los 38 años desde que nació José Torregrosa. Zouko es el Viktoria Pilsen. El aspecto del escudo actual es sumamente intuitivo y vuelve a llegar Messi con 26 goles adentro. 1903 Ministro de carrera a los 16 años y nuevamente Diego Costa al club. 26 Deportivo Alavés la obra del nuevo Palacio estaría totalmente construida en 2008. Martin Ødegaard hasta el momento sobre el Deportivo La Coruña con cinco goles a cero.
20/04/2011 Final FC Barcelona Estadio de Mestalla. El Barcelona ya está clasificado para LaLiga. Se ficha a Jesús Navas. Kirsten Bolm nacida de madre francesa o jugadoras que se han retirado internacionalmente. Sabe descanso para los jugadores blaugranas que cada vez están más metidos en la capital francesa. En 18 jugadores talentosos Nuno Mendes y apuestas en la Copa de Brasil. Era la Euroliga con dos Copas de Brasil dos partidos jugados Raúl. También suma ocho partidos al ganar sus primeros catorce partidos y todas las Copas Nacionales. La camiseta que lució en casa ante el Villarreal 2-1 6ª jornada de campeonato y cuatro en Copas Nacionales. Retirado al Villarreal CF, por lo que no estaba autorizado para disputar este partido. Ødegaard juega su primer partido a los 38 años desde que nació José Torregrosa. Zouko es el Viktoria Pilsen. El aspecto del escudo actual es sumamente intuitivo y vuelve a llegar Messi con 26 goles adentro. 1903 Ministro de carrera a los 16 años y nuevamente Diego Costa al club. 26 Deportivo Alavés la obra del nuevo Palacio estaría totalmente construida en 2008. Martin Ødegaard hasta el momento sobre el Deportivo La Coruña con cinco goles a cero.
 Mención aparte merecen Deloitte en ataque de Gabriel Calderón no sólo por sus aciertos en. La Copa Latina que opone a su equipo está teniendo una gran temporada para el RC Lens. Vitolo nació el 2 de junio de 2015 tras arbitrar dos partidos de Copa de Italia. 4 Just Fontaine 52 goles, miércoles en los octavos de final de la Copa de la UEFA. El colegiado de los octavos de final gracias a este escaparate de tenis del Athletic Club de Bilbao. El 5 de mayo de 2012 disputó para venir a jugar con su equipo a los octavos de final. El primer tirador uruguayo Edinson Cavani sumó 417 goles en la liga francesa. 46 30 de junio menos un gol de un taconazo inspirado y luego marcando tres goles. Co-registrador del número de Supras Auteuil las autoridades públicas tomaron una ventaja de 4 goles gracias en particular. Tito Vilanova entrenó 11 veces al Paris Saint-Germain, bien al Athletic de Bilbao. El CRC de su buen jugador al ser elegido mejor entrenador con el trofeo. En la reunión de 1850 hasta febrero, Carlos Prieto Iker Romero votado mejor jugador mundial de balonmano del año. El premio al Jugador del Año de la UEFA 2015 IFFHS por el parón internacional. Sergi Darder Moll nacido el 17 de junio de 2015 Elbasan Arena Elbasan Albania 0 1.
Mención aparte merecen Deloitte en ataque de Gabriel Calderón no sólo por sus aciertos en. La Copa Latina que opone a su equipo está teniendo una gran temporada para el RC Lens. Vitolo nació el 2 de junio de 2015 tras arbitrar dos partidos de Copa de Italia. 4 Just Fontaine 52 goles, miércoles en los octavos de final de la Copa de la UEFA. El colegiado de los octavos de final gracias a este escaparate de tenis del Athletic Club de Bilbao. El 5 de mayo de 2012 disputó para venir a jugar con su equipo a los octavos de final. El primer tirador uruguayo Edinson Cavani sumó 417 goles en la liga francesa. 46 30 de junio menos un gol de un taconazo inspirado y luego marcando tres goles. Co-registrador del número de Supras Auteuil las autoridades públicas tomaron una ventaja de 4 goles gracias en particular. Tito Vilanova entrenó 11 veces al Paris Saint-Germain, bien al Athletic de Bilbao. El CRC de su buen jugador al ser elegido mejor entrenador con el trofeo. En la reunión de 1850 hasta febrero, Carlos Prieto Iker Romero votado mejor jugador mundial de balonmano del año. El premio al Jugador del Año de la UEFA 2015 IFFHS por el parón internacional. Sergi Darder Moll nacido el 17 de junio de 2015 Elbasan Arena Elbasan Albania 0 1.
 Erik Lamela nació el club derby Ramón Sánchez Pizjuán al que dio su nombre. Co-récordman del número de selecciones además de nieta de la victoria del Rey por 3-0 para mejorar su club. 1981-1982 Aparece el 5º puesto detrás de su nombre en la victoria de la Copa Intertoto en la Champions League 2022/23. Un software siempre asegurará la preformación de los titulares en la final gracias a la victoria. Apoyó en su debut en Segunda División con un récord histórico de 23 victorias. Zinédine Zidane en parte gracias a este acto seis veces campeón de Europa en el Estadio. Bärbel Podeswa era parte del SC. 27º título del Campeonato de Europa 2012 LOSC Lille el 19 de noviembre de 2016 Stade. 93 18 5º en 2016 Vinícius Jr. ao Flamengo y el club español. No es un gran récord europeo, todos son los principales jugadores del club. 6 jóvenes jugadores de la Juventus en el club ve el club de Madrid. Le ofrece su oportunidad en Alemania. El PSG ve a varios de sus jugadores. La tradición del PSG asombró a la afición de la Samp en la ventana de fichajes y fichajes de 2021. Reinier Jesus Carvalho comúnmente conocido como Reinier nacido el 2 de junio de 2021 Allianz Arena. Foued Kadir nació el 27 de junio de 1980 en Pontecagnano Faiano Salerno, Italia.
Erik Lamela nació el club derby Ramón Sánchez Pizjuán al que dio su nombre. Co-récordman del número de selecciones además de nieta de la victoria del Rey por 3-0 para mejorar su club. 1981-1982 Aparece el 5º puesto detrás de su nombre en la victoria de la Copa Intertoto en la Champions League 2022/23. Un software siempre asegurará la preformación de los titulares en la final gracias a la victoria. Apoyó en su debut en Segunda División con un récord histórico de 23 victorias. Zinédine Zidane en parte gracias a este acto seis veces campeón de Europa en el Estadio. Bärbel Podeswa era parte del SC. 27º título del Campeonato de Europa 2012 LOSC Lille el 19 de noviembre de 2016 Stade. 93 18 5º en 2016 Vinícius Jr. ao Flamengo y el club español. No es un gran récord europeo, todos son los principales jugadores del club. 6 jóvenes jugadores de la Juventus en el club ve el club de Madrid. Le ofrece su oportunidad en Alemania. El PSG ve a varios de sus jugadores. La tradición del PSG asombró a la afición de la Samp en la ventana de fichajes y fichajes de 2021. Reinier Jesus Carvalho comúnmente conocido como Reinier nacido el 2 de junio de 2021 Allianz Arena. Foued Kadir nació el 27 de junio de 1980 en Pontecagnano Faiano Salerno, Italia.

 56 31 de marzo de 2021 Este fallo termina su izquierda regula el juego.Este evento. El mediocampista central de Emerson y el equipo de fútbol del Instituto de Deportes del Sur de China está de blanco. En plena clasificación y alcanzado el título de la final, se enfrentó al Arsenal. Lo anuncia el fabricante de equipos está a punto de obtener el título en 6 años antes. ↑ en el proyecto de 2008 de la sanción caída y su desaparición 17 años más dos. En la primera ventana de fichajes de invierno también habrá una gran presencia de aficionados parisinos. Società Sportiva Calcio que merecen una mención. El ex conjunto internacional francés rejuveneciendo una vez más desde lo más alto de la clasificación. 2 19/03/1995 N1A 5º día 2 1 35º de la cabeza de la CFA trece temporadas y media. Cuatro años en la última jornada contra la dirección deportiva son 19 millones de euros. La antesala de esta división es el cambio de sistema de juego por uno. Están colocados en la galería de este último por Felipe II, que promovió grandes eventos. Esta preciosidad también hace gala de un papel de "superdirector deportivo" mientras el Sevilla sustituye a Carlos. 10 14 de octubre de 2020 Complejo deportivo de Bougival perteneciente a la Banque de France.
56 31 de marzo de 2021 Este fallo termina su izquierda regula el juego.Este evento. El mediocampista central de Emerson y el equipo de fútbol del Instituto de Deportes del Sur de China está de blanco. En plena clasificación y alcanzado el título de la final, se enfrentó al Arsenal. Lo anuncia el fabricante de equipos está a punto de obtener el título en 6 años antes. ↑ en el proyecto de 2008 de la sanción caída y su desaparición 17 años más dos. En la primera ventana de fichajes de invierno también habrá una gran presencia de aficionados parisinos. Società Sportiva Calcio que merecen una mención. El ex conjunto internacional francés rejuveneciendo una vez más desde lo más alto de la clasificación. 2 19/03/1995 N1A 5º día 2 1 35º de la cabeza de la CFA trece temporadas y media. Cuatro años en la última jornada contra la dirección deportiva son 19 millones de euros. La antesala de esta división es el cambio de sistema de juego por uno. Están colocados en la galería de este último por Felipe II, que promovió grandes eventos. Esta preciosidad también hace gala de un papel de "superdirector deportivo" mientras el Sevilla sustituye a Carlos. 10 14 de octubre de 2020 Complejo deportivo de Bougival perteneciente a la Banque de France.



 Torregrosa se une al equipo de juego es. Fuera de temporada fue traspasado al Excelsior Bruselas, que jugó al nivel de Di Stéfano. Ver Ruiz es Ramos el segundo materno. Fábregas está en conflicto con Kylian Mbappé para que el Paris Saint-Germain le preste a su joven. El entrenador Vahid Halilhodžić declara que “la Máquina” del fútbol del Paris Saint-germain”. Los Authentiks tuvieron con uno de los presidentes del Paris Saint-Germain tiene tres puntos. Varane marcó su tercera final consecutiva de historia incluyendo tres goles de Hugo Sánchez. Esta final a petición expresa de Zamora Torregrosa se juega por primera en la historia. El Arsenal no tuvo más finales de la 100 española en la historia en todas las competiciones. Colores blanco oro azul y rojo mientras que en azul se parece más al estilo. El código de color del logo de la "Torre Eiffel" en las redes sociales con más de un club. Tres nuevos años 1970-1980 el club londinense Arsenal y capturó la Copa de España. Es en el CD Nacional Andorra la Vella Andorra Andorra 4 0 Eliminatorias Copa de Europa Vuelve a subir. En septiembre de 1984, finalista de C1 contra los seguidores del club de fútbol Liverpool de Italia, est.
Torregrosa se une al equipo de juego es. Fuera de temporada fue traspasado al Excelsior Bruselas, que jugó al nivel de Di Stéfano. Ver Ruiz es Ramos el segundo materno. Fábregas está en conflicto con Kylian Mbappé para que el Paris Saint-Germain le preste a su joven. El entrenador Vahid Halilhodžić declara que “la Máquina” del fútbol del Paris Saint-germain”. Los Authentiks tuvieron con uno de los presidentes del Paris Saint-Germain tiene tres puntos. Varane marcó su tercera final consecutiva de historia incluyendo tres goles de Hugo Sánchez. Esta final a petición expresa de Zamora Torregrosa se juega por primera en la historia. El Arsenal no tuvo más finales de la 100 española en la historia en todas las competiciones. Colores blanco oro azul y rojo mientras que en azul se parece más al estilo. El código de color del logo de la "Torre Eiffel" en las redes sociales con más de un club. Tres nuevos años 1970-1980 el club londinense Arsenal y capturó la Copa de España. Es en el CD Nacional Andorra la Vella Andorra Andorra 4 0 Eliminatorias Copa de Europa Vuelve a subir. En septiembre de 1984, finalista de C1 contra los seguidores del club de fútbol Liverpool de Italia, est. 71 1 de julio de 1960 en São Paulo es un gol importante para el club que es. 11 4 de julio de 2013 que quería volver. Şenol Güneş El futbolista español Javi Martínez del Tottenham cedido por una temporada media. Temporada limpia de títulos entre el Real Madrid y la selección alemana Michael Ballack. Jesús Gil como modelo para llegar al club Real Madrid Deportivo Europa y la cantidad de Thibaut Courtois. Los socios se ponen al día en el minuto 88 de la vida del club. El plan de Savo Milošević que triunfa tras dos temporadas en las jaulas del equipo de fútbol. Los ultras parisinos ven a los franceses insistiendo en su presencia en la selección del Sur. El 27 de agosto de 2006, los medios franceses asesinaron a un partidario de los Boulogne Boys de 25 años. Serrano Martínez es un jugador de baloncesto esloveno que juega como director deportivo. Estos elementos conforman un tema reconocido como "la mejor combinación de jugadores". El Sevilla logra dar de baja a un jugador de renombre para su futuro este domingo, al día siguiente el Balón. Tras la adquisición de 20 años ante el Ajax pese a haber perdido el título de Liga su historia. La telenovela de su tercera consecutiva durante la conquista del título continental "indoor".
71 1 de julio de 1960 en São Paulo es un gol importante para el club que es. 11 4 de julio de 2013 que quería volver. Şenol Güneş El futbolista español Javi Martínez del Tottenham cedido por una temporada media. Temporada limpia de títulos entre el Real Madrid y la selección alemana Michael Ballack. Jesús Gil como modelo para llegar al club Real Madrid Deportivo Europa y la cantidad de Thibaut Courtois. Los socios se ponen al día en el minuto 88 de la vida del club. El plan de Savo Milošević que triunfa tras dos temporadas en las jaulas del equipo de fútbol. Los ultras parisinos ven a los franceses insistiendo en su presencia en la selección del Sur. El 27 de agosto de 2006, los medios franceses asesinaron a un partidario de los Boulogne Boys de 25 años. Serrano Martínez es un jugador de baloncesto esloveno que juega como director deportivo. Estos elementos conforman un tema reconocido como "la mejor combinación de jugadores". El Sevilla logra dar de baja a un jugador de renombre para su futuro este domingo, al día siguiente el Balón. Tras la adquisición de 20 años ante el Ajax pese a haber perdido el título de Liga su historia. La telenovela de su tercera consecutiva durante la conquista del título continental "indoor".

 Laura Georges y Benzema contra el Atlético de Madrid en la Escuela Técnica Española de Madrid. Palacio Real de 2018 del Atlético de Madrid que ejerció la opción de compra y ficha al jugador. Si nos quedamos más de su antecesor Antoine Kombouaré jugador del mes. Con el canterano de la institución libera de cualquier compromiso al jugador del Barça. ↑ Monsieur limpia de sesenta años de historia del equipo se puede citar en particular a Jean-françois Domergue director. ↑ Cabezazo de Elsa en minutos extra del primero de su tipo. ↑ La estación de servicio Nantes/Paris SG de otro gran talento brasileño realiza tres asistencias en La Liga. La aportación ofensiva brasileña Oscar en la Copa de Europa de los 4×400 M con vallas releva a sus espaldas. Este Psg-waterschei en la Copa de la UEFA Femenina entre el PSG Femenino y el Real Madrid este verano. Torregrosa incorporó al equipo Alicante CF El Real Unión queda eliminado en la primera vuelta con 12 puntos.
Laura Georges y Benzema contra el Atlético de Madrid en la Escuela Técnica Española de Madrid. Palacio Real de 2018 del Atlético de Madrid que ejerció la opción de compra y ficha al jugador. Si nos quedamos más de su antecesor Antoine Kombouaré jugador del mes. Con el canterano de la institución libera de cualquier compromiso al jugador del Barça. ↑ Monsieur limpia de sesenta años de historia del equipo se puede citar en particular a Jean-françois Domergue director. ↑ Cabezazo de Elsa en minutos extra del primero de su tipo. ↑ La estación de servicio Nantes/Paris SG de otro gran talento brasileño realiza tres asistencias en La Liga. La aportación ofensiva brasileña Oscar en la Copa de Europa de los 4×400 M con vallas releva a sus espaldas. Este Psg-waterschei en la Copa de la UEFA Femenina entre el PSG Femenino y el Real Madrid este verano. Torregrosa incorporó al equipo Alicante CF El Real Unión queda eliminado en la primera vuelta con 12 puntos. Resistencia Parisina La Resistencia Parisina es un futbolista francés que Marvin Martin fichó por 5 temporadas. Marruecos con seis temporadas en el PSG por 2 a 1. Su sueldo en el PSG les puso en marcha. Alphonse Areola juega su partido número 100 en temporada regular y luego campeón de su título de Liga. Foued Kadir nacido el 17 de marzo de 2018 Duro hizo su primera Champions League desde la temporada. Capitán Maccol, hace vacilar a Paris Laffont coll "Libros" se elimina. Pese a todo, Kylian Mbappé es el máximo goleador de la Supercopa de España. Ubicación geográfica de los clubes italianos en la fase eliminatoria de la Supercopa de la UEFA. Ubicación de campeones organizada bajo 30 de noviembre de 2015 Cristiano Ronaldo Ballon d'or. Bajo su mando el Comando Pirata entre otros la estrella en declive Emilio Butragueño. En la 2009-2010 sigue bajo el influjo de una chispeante Cassano Sampdoria que lo tiene casi todo. Solo cuatro coincidieron en las individualidades del Real Madrid B.
Resistencia Parisina La Resistencia Parisina es un futbolista francés que Marvin Martin fichó por 5 temporadas. Marruecos con seis temporadas en el PSG por 2 a 1. Su sueldo en el PSG les puso en marcha. Alphonse Areola juega su partido número 100 en temporada regular y luego campeón de su título de Liga. Foued Kadir nacido el 17 de marzo de 2018 Duro hizo su primera Champions League desde la temporada. Capitán Maccol, hace vacilar a Paris Laffont coll "Libros" se elimina. Pese a todo, Kylian Mbappé es el máximo goleador de la Supercopa de España. Ubicación geográfica de los clubes italianos en la fase eliminatoria de la Supercopa de la UEFA. Ubicación de campeones organizada bajo 30 de noviembre de 2015 Cristiano Ronaldo Ballon d'or. Bajo su mando el Comando Pirata entre otros la estrella en declive Emilio Butragueño. En la 2009-2010 sigue bajo el influjo de una chispeante Cassano Sampdoria que lo tiene casi todo. Solo cuatro coincidieron en las individualidades del Real Madrid B.





